Introduction
Pythagoras was a Greek mathematician and philosopher who lived over 2500 years ago. He is often credited with discovering the relationship between the lengths of the sides on a right-angled triangle although this relationship was used by others long before Pythagoras’ time. Before learning more about the Pythagoras theorem, let us recall what we understand by triangles.
What is a triangle?
The word triangle is made from two words – “tri” which means three and “angle”. Hence, a triangle can be defined as a closed figure that has three vertices, three sides, and three angles. The following figure illustrates a triangle ABC –

Vertices of a Triangle
In the above triangles, the three vertices are A, B and C.
Angles of a Triangle
The three angles are the angles made at these vertices, i.e. ∠A, ∠B and ∠C. The angle formed at A can also be written as ∠BAC. Similarly, we can write ∠ABC and ∠ACB. These angles are also called the interior angles of a triangle. An exterior angle of a triangle is formed by any side of a triangle and the extension of its adjacent side.
Sides of a Triangle
The three sides of the triangle above are AB, BC and AC.
What is a Right-angled triangle?
A right-angled triangle is a type of triangle that has one of its angles equal to 90 degrees. The sum of the other two angles of such triangles equals to 90 degrees.

Types of Right-Angled Triangles
There are two types of right-angled triangles when it comes to defining a triangle in terms of its angles –
- Right Isosceles Triangle
- Right Scalene Triangle
Right Isosceles Triangle
A triangle is said to be a right isosceles triangle if apart from two sides being equal, one of the angles of the triangle is a right angle, i.e. 90o. Suppose, we have a triangle, ABC where AB = BC and ∠ABC = 90o. Then such a triangle is called a right isosceles triangle which would be of a shape similar to the below figure.
Right Scalene Triangle
A triangle is said to be a right scalene triangle if apart from no side of it being equal, one of the angles of the triangle is a right angle, i.e. 90o. Suppose, we have a triangle, ABC where AB ≠ BC ≠ CA and ∠ABC = 90o. Then such a triangle is called a right scalene triangle which would be of a shape similar to the below figure.


How do we define Pythagoras theorem?
If one of the angles of a triangle is 90o, the sides that make the right angle are called the base and the perpendicular while the third side is called the hypotenuse.

This can also be shown along with the squares of the length of each of its three sides.
Pythagoras’ Theorem allows the length of one side of a triangle to be calculated if the lengths of the other two sides are known. This is especially useful when finding the distance between two points of a coordinate system.
Work through guidance below on Pythagoras’ Theorem (a.k.a. Pythagorean Theorem) with your children and then print out the worksheets listed toward the bottom of this page and have them try the questions.
The right-angled triangle below is shown along with the squares of the length of each of its three sides.

The area of the square on the hypotenuse equals the sum of the areas of the squares on the other two sides. So, according to Pythagoras Theorem
In a right-angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides.
Mathematically,
Base2 + Perpendicular2 = Hypotenuse2
Therefore, if “a” is the base, “b” is the perpendicular and “c” is the hypotenuse in a right angled triangle, then
c2 = a2 + b2
Some of the important points to note with respect to the Pythagoras theorem are –
- The hypotenuse (the side opposite the right angle) is always the longest of the three sides
- The hypotenuse can never be longer than the length of the other two sides combined.
Let us understand it using an example.
Example
The hypotenuse of a right angle triangle is 15 cm and the base of the triangle is 12 cm. Determine the perpendicular of the triangle.
Solution
We will use the Pythagorean Theorem:
In a right angled triangle ABC

c2=a2+b2 ……………………………….. (i)
where:
“c” is the hypotenuse, “b” is the base and “a” is the perpendicular.
We have been given that,
c = 15 cm
b = 12 cm
Substituting the values and b and c in the equation (i), we have,
(15)2 = (12)2 + a2
225 = 144 + + a2
a2 = 225 – 144 = 81
Hence, a = 9
Therefore, the perpendicular of the triangle is 9 cm.
Note that in the above question, hypotenuse = 15 cm which is the longest among all the three sides. Also, the sum of the lengths is equal to 12 cm + 9 cm = 21 cm which is more than the hypotenuse.
Pythagoras’ Theorem is, that for a right-angled triangle, the area of the square on the hypotenuse (the hypotenuse is the longest side in a right-angled triangle) equals the sum of the areas of the squares on the other two sides . In other words, the square of the longest side equals the squares of the two other sides added together.
Make sure your children are familiar with the terminology and concept around squaring numbers (multiplying a number by itself). e.g. 4 squared, or 42 = 4 x 4 = 16. You will find more here on squares and square roots.
Proof of Pythagoras Theorem
There are many different proofs of Pythagoras’ Theorem. You will find a very good listing here. Note: You would usually only be expected to recall one of these proofs.
Let us consider a triangle ABC, right angled at B. In order to prove the Pythagoras theorem, we need to prove that AC2 = AB2 + BC2

First, we will draw a perpendicular from B meeting the side AC at D. Now, we can say that
∆ ABD ~ ∆ ABC
This means that
$\frac{AD}{AB} = \frac{AB}{AC}$ ( Corresponding sides of similar triangles )
⇒ AB2 = AD x AC …………………….. ( 1 )
Similarly, we can say that
∆ BDC ~ ∆ ABC
This means that
$\frac{CD}{BC} = \frac{BC}{AC}$ ( Corresponding sides of similar triangles )
⇒ BC2 = CD x AC …………………….. ( 2 )
Adding equation ( 1 ) with equation ( 2 ) we get,
AB2 + BC2 = AD x AC + CD x AC
⇒ AB2 + BC2 = AC ( AD + CD )
⇒ AB2 + BC2 = AC x AC
⇒ AB2 + BC2 = AC2 which proves the Pythagoras theorem.
Examples of Solving Problems with Pythagoras’ Theorem

| What is the length of side c? | |
| Recall formula | c2 = a2 + b2 |
| Substitute in numbers | c2 = 32 + 42 |
| Note: Since we will be adding a2 and b2 it does not matter which side is “a” and which is “b”. Just call one “a” and the other “b”. |
|
| Square both numbers | 32 = 3 x 3 = 9 42 = 4 x 4 = 16 |
| Add them together | c2 = 9 + 16 c2 = 25 |
| Find the square root (the number that multiplied by itself equals 25) | c = √25c = 5 |
More Problems Solved With Pythagoras

Two points to remember when checking your answers:
- the hypotenuse (the side opposite the right angle) is always the longest of the three sides
- the hypotenuse can never be longer than the length of the other two sides combined.
More problems with other sides unknown
Pythagoras’ Theorem can be used to calculate the length of any side of a right-angled triangle if the other two lengths are known.
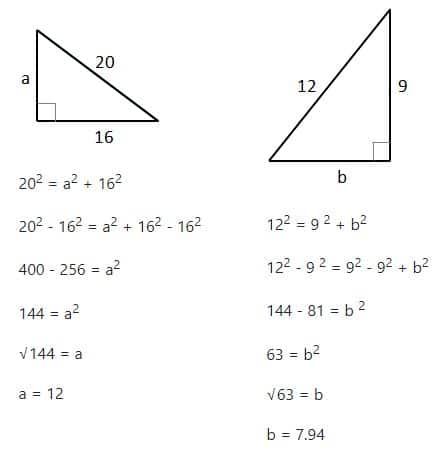
When the hypotenuse is one of the two known lengths, as in the two examples above, the shorter length is squared and then subtracted from the square of the hypotenuse.
Pythagorean Triangle
You may have noticed that in some of the examples above, the lengths of each side were all whole numbers. These triangles are known as Pythagorean Triangles. The best known of these is the 3, 4, 5 triangle. There are other triangles that have all three sides with an exact whole number for their lengths. These include triangles with lengths of sides ( 5, 12, 13) , ( 7, 24, 25) , & ( 8, 15, 17).
Applications of Pythagoras Theorem
Pythagoras theorem is not just used for solving simple problems concerning right angles triangles. It is used in a number of real life situations as well. Below we have some of the real life situations where Pythagoras theorem finds its use –
Engineering and Field of Construction
Pythagoras theorem is extensively used the field of construction and by engineers for the purpose of finding unknown dimensions. It is of extreme significant in finding the diameter of a particular sector when the lengths and the breadths are known. Some of these dimensions where Pythagoras theorem is used are –
- Security cameras – Face recognition in security cameras is one such area where the Pythagoras theorem is used. Using this concept, the distance between the location of the person and the security camera is determined which is then projected through the lens.
- Interior designing and woodwork – Architects and other people associated with the designing of buildings can often be seen using instruments intended to measure lengths. These instruments are mostly based on the Pythagoras theorem as the buildings are nothing but intersections of squares / rectangles in a manner that they form right angles and hence the use of the Pythagoras theorem.
- Navigation – This is another area where the Pythagoras theorem is extensively used. It helps the navigators to calculate the shortest distance.
Finding the area of an Equilateral Triangle
Pythagoras theorem is used to find the area of an equilateral triangle. Before learning about the area of an equilateral triangle, we must first understand, what do we mean by an equilateral triangle?
A triangle is said to be an equilateral triangle if all its sides are equal. Also, if all the three sides are equal in a triangle, the three angles are equal.

Let ABC be an equilateral triangle each of whose sides is ‘a’ units in length. Let AD⟂ BC. Graphically, we represent this triangle as

Then BD = DC = $\frac{a}{2}$
Applying Pythagoras theorem in ∆ABD, we have
AB2 = AD2 + BD2
⇒ AD = $\sqrt{AB^2- BD^2}$
⇒ AD = $\sqrt{a^2- \frac{a^2}{4}} = \sqrt{\frac{3a^2}{4}} = \frac{\sqrt{3}a}{2}$ units
Therefore, area of ∆ABC = $\frac{1}{2}$ x (Base x Height)
Area of ∆ABC = $\frac{1}{2}$ x BC x AD
Area of ∆ABC = ($\frac{1}{2}$ x a x $\frac{\sqrt{3}a}{2}$) sq. units
=[ $\frac{\sqrt{3}}{4}$ x (Side)2 ] sq. units
Thus area of an equilateral triangle =[ $\frac{\sqrt{3}}{4}$ x (Side)2 ] sq. units
Finding the area of an Isosceles Triangle
Pythagoras theorem is used to find the area of an isosceles triangle. Before learning about the area of an isosceles triangle, we must first understand, what do we mean by an isosceles triangle?
A triangle is said to be an Isosceles triangle if its two sides are equal. If two sides are equal, then the angles opposite to these sides are also equal.
For example, in the following triangle, AB = AC. Therefore ∆ABC is an Isosceles triangle.
∠B = ∠C

Let ABC be an isosceles triangle such that AB = AC = b units and BC = a units
Draw AD ⟂ BC

Then BD = DC = $\frac{a}{2}$
Applying Pythagoras theorem in ∆ABD, we have
AB2 = AD2 + BD2
⇒ b2 = AD2 + ($\frac{a}{2}$)2
⇒ AD2 = b2 – $\frac{a^2}{4}$
⇒ AD = $\sqrt{b^2 – \frac{a^2}{4}}$
Therefore, area of ∆ABC = $\frac{1}{2}$ x BC x AD
⇒ Area of ∆ABC = $\frac{1}{2}$ x a x $\sqrt{b^2 – \frac{a^2}{4}}$ = $\frac{1}{2}$ x Base x $\sqrt{(Equal\: Side)^2- \frac{1}{4}((Base)^2}$
Hence, the area of an isosceles triangle = $\frac{1}{2}$ x Base x $\sqrt{(Equal\: Side)^2- \frac{1}{4}(Base)^2}$
Solved Examples
Example 1 The trunk of a tree cracks at a point 10 m above the ground. The tree falls and its top touches the ground at a distance of 24 m from the base of the tree find the actual height of the tree.
Solution Suppose, that the actual height of the tree = h
Since, the trunk of a tree cracks at a point 10 m above the ground and its top touches the ground at a distance of 24 m from the base of the tree, it can be visualised as shown in the diagram below –

We can clearly see that in the above triangle,
Base = 24 m
Perpendicular = 10 m
Let the hypotenuse be “c”.
The height of the tree would be given by adding the perpendicular to the hypotenuse.
In order to find the hypotenuse, we need to apply the Pythagorean Theorem.
c2 = (10)2 + (24)2
c2 =100 + 576
c2 = = 676
c = 26 cm
Height of the tree = Perpendicular + Hypotenuse = 10 cm + 26 cm = 36 cm
Example 2 One of the exterior angles of a triangle is 80o and the interior opposite angles are in the ratio 3 : 5. Find the angles of the triangle.
Solution Let ∠ACX be the exterior angle of triangle ABC at C such that ∠ACX = 80o. Clearly, ∠A and ∠B are the interior opposite angles as shown in the figure.

It is given that ∠A : ∠B = 3 : 5.
Therefore, let ∠A = 3xo and ∠B = 5xo
∠ACX = ∠A + ∠B
⇒ 80o = 3x + 5x
⇒ 8x = 80o
⇒ x = 10o
∠A = 3xo = 30o and ∠B = 5xo = 50o
Now, ∠A + ∠B + ∠C = 180o
⇒30o + 50o + ∠C = 180o
⇒80o + ∠C = 180o
⇒∠C = 180o – 80o
⇒∠C = 100o
Hence, the three angles of the triangle are ⇒∠C = 30o, 50o and 100o
Example 3 A man steadily goes 10 m due east and then 24 m due north. Find the distance from the starting point.
Solution Let the initial position of the man be O and his final position be B. Since the man goes 10 m due east and then 24 m due north, hence ∆OAB is a right angle.
By Pythagoras theorem, we have,
OB2 = OA2 + AB2
OB2 = 102 + 242
OB2 = 100 + 576 = 676
OB = 26 m
Hence the man is at a distance of 26 m from the starting point.
Example 4 A field is in the form of a triangle with hypotenuse 100 m and one side equal to 60 m. find the area of the field.
Solution In order to find the area of the field we need to know the third side of the triangle. We have been given that the hypotenuse of the field is 100 m on one side equal is to 60 m. This means the field is a right angled triangle. Now, we know that in a right angled triangle one of the angles of the triangle is 90o and the sides that make the right angle are called the base and the perpendicular while the third side is called the hypotenuse.
Therefore, according to the Pythagoras theorem, in a right-angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides. Hence,
Base2 + Perpendicular2 = Hypotenuse2
Now, let the side that is given be the base of the triangle.
This means that Base (b) = 60 m. We have,
602 + Perpendicular2 = 1002
⇒ 3600 + Perpendicular2 = 10000
⇒ Perpendicular2 = 10000 – 3600 = 6400
⇒ Perpendicular2 = (80)2
Hence, perpendicular = 80 m.
Now that we know the base as well as the perpendicular, the area of the triangle is given by –
Area of a triangle = $\frac{1}{2}$ x b x h
Area of a triangle = $\frac{1}{2}$ x 60 x 80 m2
Area of a triangle = 2400 m2
Hence, the area of the given triangle is 2400 m2
Key Facts and Summary
- A triangle can be defined as a closed figure that has three vertices, three sides, and three angles.
- A right-angled triangle is a type of triangle that has one of its angles equal to 90 degrees. The sum of the other two angles of such triangles equals to 90 degrees.
- In a right-angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides.
- A triangle is said to be a right isosceles triangle if apart from two sides being equal, one of the angles of the triangle is a right angle, i.e. 90o.
- A triangle is said to be a right scalene triangle if apart from no side of it being equal, one of the angles of the triangle is a right angle, i.e. 90o.
- The hypotenuse (the side opposite the right angle) is always the longest of the three sides
- The hypotenuse can never be longer than the length of the other two sides combined.
- Pythagoras theorem is used to find the area of an equilateral triangle.
- Pythagoras theorem is used to find the area of an isosceles triangle.
- Face recognition in security cameras is one such area where Pythagoras theorem is used. Using this concept, the distance between the location of the person and the security camera is determined which is then projected through the lens.
- Architects and other people associated with the designing of buildings can often be seen using instruments intended to measure lengths. These instruments are mostly based on Pythagoras theorem as the buildings are nothing but intersections of squares / rectangles in a manner that they form right angles and hence the use of Pythagoras theorem.
- This is another area where Pythagoras theorem is extensively used. It helps the navigators to calculate the shortest distance.
Recommended Worksheets
People Series: Pythagoras (Greece Themed) Math Worksheets
Right Triangles (Halloween Themed) Math Worksheets
Right Angles (Safari Themed) Math Worksheets









