Isosceles Triangle

Triangles

The word triangle is made from two words – “tri” which means three and “angle”. Hence, a triangle can be defined as a closed figure that has three vertices, three sides, and three angles. The following figure illustrates a triangle ABC –

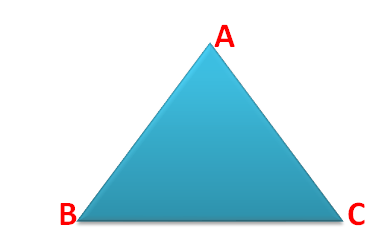
Vertices of a Triangle 

In the above triangles, the three vertices are A, B and C. 

Angles of a Triangle

The three angles are the angles made at these vertices, i.e. ∠A, ∠B and ∠C.  The angle formed at A can also be written as ∠BAC. Similarly, we can write ∠ABC and ∠ACB. These angles are also called the interior angles of a triangle. An exterior angle of a triangle is formed by any side of a triangle and the extension of its adjacent side.

Sides of a Triangle

The three sides of the triangle above are AB, BC and AC.

What is an Isosceles Triangle?

The term isosceles triangle is derived from the Latin word ‘īsoscelēs’, and the ancient Greek word ‘ἰσοσκελής (isoskelḗs)’ which means “equal-legged”. Also, ancient Babylonian and Egyptian mathematicians were of the know-how on the calculations required to find the ‘area’ much before the ancient Greek mathematicians started studying the isosceles triangle. 

Now, let us understand the definition of an isosceles triangle.

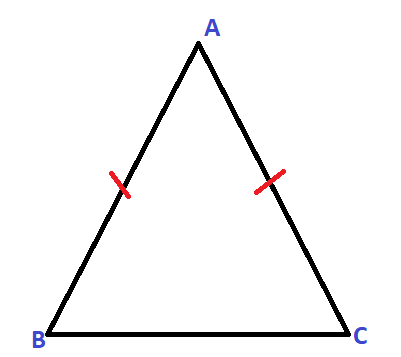
A triangle is said to be an Isosceles triangle if its two sides are equal. If two sides are equal, then the angles opposite to these sides are also equal.

For example, in the following triangle, AB = AC. Therefore ∆ABC is an Isosceles triangle. 

Since AB = AC

 ∠B = ∠C

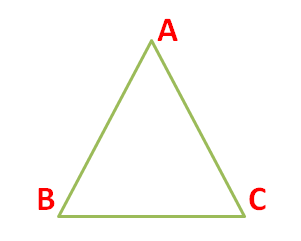
Based on the interior angles, the isosceles triangle can further be divided into the following three types – 

Right Isosceles Triangle

A triangle is said to be a right isosceles triangle if apart from two sides being equal, one of the angles of the triangle is a right angle, i.e. 90^o_. Suppose, we have a triangle, ABC where AB = BC and ∠ABC = 90^o.  Then such a triangle is called a right isosceles triangle which would be of a shape similar to the below figure.

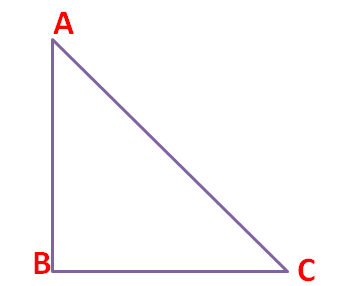
Acute Isosceles Triangle

A triangle is said to be an acute isosceles triangle if apart from two sides being equal, all the three interior angles of the triangle are acute angles, i.e. all the three angles are less than 90^o. Suppose, we have a triangle, ABC where AB = BC and all the three angles are acute angles.  Then such a triangle is called an acute isosceles triangle which would be of a shape similar to the below figure. 

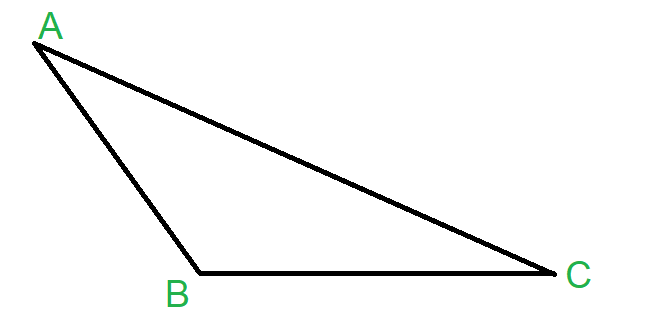
Obtuse Isosceles Triangle

A triangle is said to be an obtuse isosceles triangle if apart from two sides being equal, one of the angles of the triangle is an obtuse angle, i.e. greater than 90^o_. Suppose, we have a triangle, ABC where AB = BC and ∠ABC > 90^o.  Then such a triangle is called an obtuse isosceles triangle which would be of a shape similar to the below figure.

Properties of Isosceles Triangles

Properties of Isosceles triangles can be stated as under – 

• Sides, Angles and Vertices

We already know that an Isosceles triangle always has exactly three sides and three vertices. This is the basic property of any triangle. 

• Angle Sum Property of an Isosceles Triangle

The sum of the measure of the three interior angles of an Isosceles triangle is always 180^o.

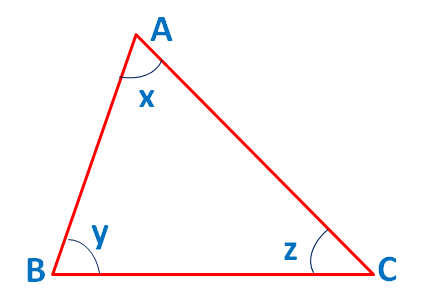
In the above triangle, ∠x + ∠y + ∠z = 180^o

• Triangle Inequality Property

According to the triangle inequality property, the sum of two sides of an Isosceles triangle is always greater than or equal to the third side. If this sum is less than the third side, it is not possible to construct the triangle. For example, in a triangle ABC,

AB + BC ≥ AC

BC + AC ≥ AB

AB + AC ≥ BC

• Pythagoras Theorem

If one of the angles of a triangle is 90^o, the sides that make the right angle are called the base and the perpendicular while the third side is called the hypotenuse.

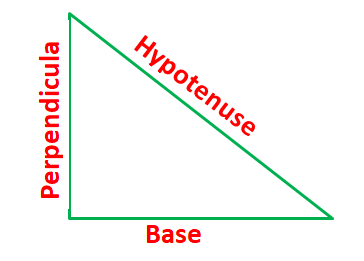
According to Pythagoras Theorem

In a right-angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides.

Mathematically,

Base² + Perpendicular² = Hypotenuse²

Therefore, if “a” is the base, “b” is the perpendicular and “c” is the hypotenuse in a right angled triangle, then

c² = a² + b²

• Exterior Angle Property

According to this property, the exterior angle of an Isosceles triangle is always equal to the sum of the interior opposite angles.

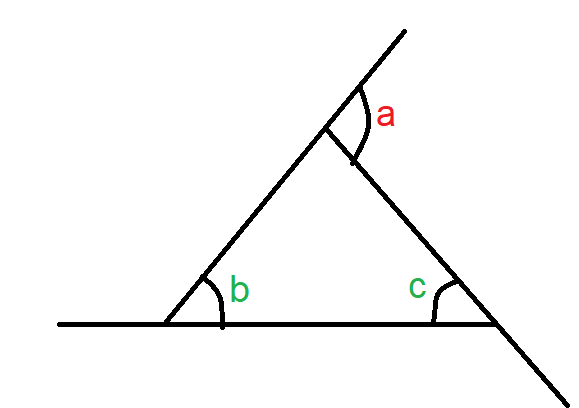
For example, in the above triangle, the exterior angle a equals the sum of the interior angles b and c.

∠a = ∠b + ∠c

Area of an Isosceles Triangle

Before we learn how to find the area of an isosceles triangle, we must first understand what we mean by the area of a triangle?

We must recall that a triangle is a polygon that is made of three edges and three vertices. The vertices join together to make three sides of a triangle. The area occupied between these three sides is called the area of a triangle.

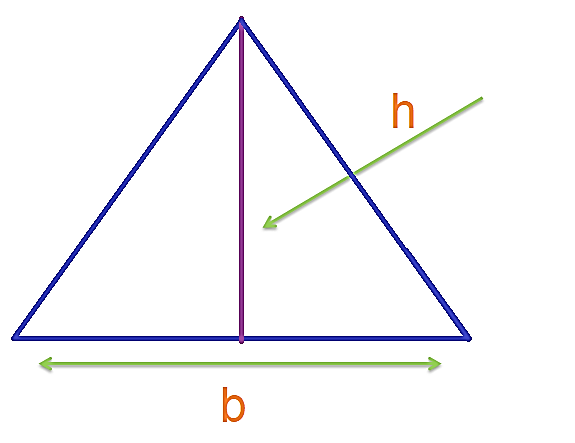
In general, the area of an Isosceles triangle is defined by $\frac{1}{2} x b x h$

Area of a triangle = $\frac{1}{2} x b x h$

where b = base of the triangle (or any one side of the triangle), and  

h = Height of the triangle from that base (or side)

The following figure illustrates the base and the height of a triangle

The area of an isosceles triangle can be calculated using the general formula as stated above. But, can we calculate the area of an isosceles triangle if we know the two equal sides and the base? Let us find out.

Area of an Isosceles Triangle when the two Equal Sides and the Base is known

Let ABC be an isosceles triangle such that AB = AC = b units and BC = a units

Draw AD ⟂ BC 

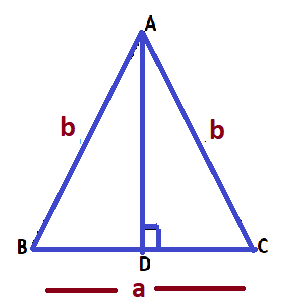
Then BD = DC = $\frac{a}{2}$

Applying Pythagoras theorem in ∆ABD, we have

AB² = AD² + BD²

⇒ b² = AD² + $(\frac{a}{2})^2$

⇒ AD² = b² – $\frac{a^2}{4}$

⇒ AD = $\sqrt{b^2 – \frac{a^2}{4}}$

Therefore, area of ∆ABC = $\frac{1}{2}$ x BC x AD

⇒ Area of ∆ABC = $\frac{1}{2}\:x\:a\:x \sqrt{b^2 – \frac{a^2}{4}} = \frac{1}{2}\:x\:Base\:x\: \sqrt{(Equal\:Side)^2 – \frac{1}{4}((Base)^2}$

Hence, the area of an isosceles triangle = $\frac{1}{2}\:x\:Base\:x\: \sqrt{(Equal\:Side)^2 – \frac{1}{4}((Base)^2}$

Now, let us learn how to find area of a right angled isosceles triangle.

Area of a Right angled Isosceles Triangle

From the definition of a right angled isosceles triangle that we have learnt above, it can be seen that a right angled isosceles triangle has a defined altitude in the form of the side AB and the side BC is its base. Hence the area of a right angled isosceles triangle can be calculated using the general formula only, which is given by

Area of a triangle = $\frac{1}{2}$ x b x h

where b = base of the triangle, and h = Height of the triangle from that base (or side)

Let us now learn about the Isosceles Triangle theorem that is related to finding the area of an isosceles triangle. 

Isosceles Triangle Theorem

Isosceles triangle theorem states that “In an isosceles triangle, the angles opposite to the equal sides are equal. Conversely, if the base angles of a triangle are equal, then the triangle is isosceles.”

Let us understand the above theorem by an example.

Consider an isosceles triangle △ ABC, with AB = AC.  Draw a bisector AD of ∠A which meets BC in D.

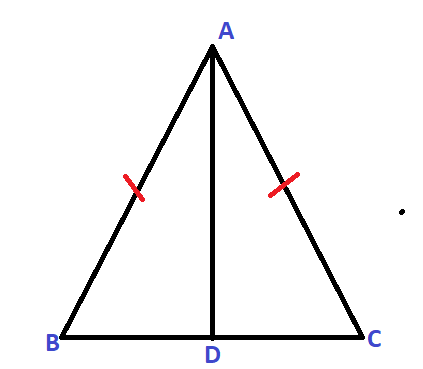
Now, we have,

AB =  AC

∠ BAD =  ∠ CAD

AD = AD 

Hence, by SAS criterion of congruence, we have

∆ ABD ≅ ∆ ACD

This further implies that ∠ B =  ∠ C [ This is due to the rule that Corresponding parts of congruent triangles are equal ] 

Now, that we have learnt how to find the area of an isosceles triangle, let us see how to find its perimeter.

Perimeter of a Triangle

The sum of the lengths of the sides of the isosceles triangle is called its perimeter.  If the lengths of the sides of an isosceles triangle are a, a and b units, then,

Perimeter of the Isosceles Triangle = a + a + b = 2a + b units. 

Let us understand this by an example.

Suppose we want to find the perimeter of the following triangle – 

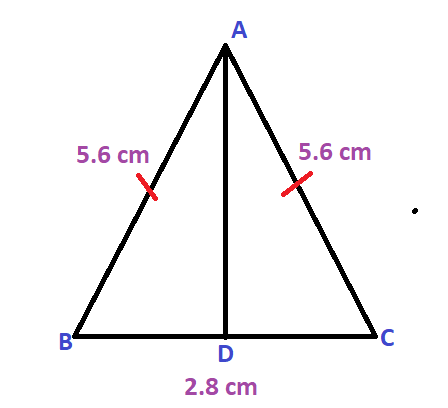
In the above figure, we can clearly see that two of the sides of the triangle are equal. This means that the given triangle is an isosceles triangle. Now the perimeter of the triangle is given by the sum of all its sides. Hence, 

Perimeter of the given triangle = 5.6 cm + 5.6 cm + 2.8 cm = 14 cm.

Hence, the perimeter of the given triangle = 14 cm

Let us now learn about some other theorems related to isosceles triangles.

Theorems of Isosceles Triangles

1. The Isosceles Decomposition Theorem –  In an isosceles triangle, if a line segment goes from the vertex angle to the base, the following conditions are equivalent:
   1. The line segment meets the base at its midpoint,
   2. The line segment is perpendicular to the base.
   3. The line segment bisects the vertex angle.
2. If the bisector of an angle in a triangle is perpendicular to the opposite side, the triangle is isosceles.
3. If the line from an angle of a triangle that is perpendicular to the opposite side meets the opposite side at its midpoint, then the triangle is isosceles. 
4. If the bisector of an angle in a triangle meets the opposite side at its midpoint, then the triangle is isosceles.
5. A point is on the perpendicular bisector of a line segment if and only if it lies the same distance from the two endpoints.

Solved Examples

Example 1 One of the angles of a triangle is 80^o and the other two angles are equal. Find these angles.

Solution Since the other two angles are equal, let each of these angles be x. By angle sum property, the sum of the measure of the three interior angles of a triangle is always 180^o.

Therefore,

x +x + 80^o = 180^o

2x +80^o = 180^o

2x = 100^o

x = 50^o

The angles each measure 50^o

Example 2 Find the value of x in the following figure –

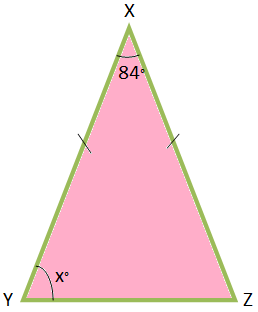
Solution We have been given an isosceles triangle, where x y = x z.

Now, we know that by the angle sum property of a triangle, the sum of three angles of a triangle is always equal to 180^o.

Therefore, we can say that

∠ x + ∠ y  + ∠ z = 180^o

Now, since xy =  xz, therefore, by isosceles traingel tehorem,

∠ y   = ∠ z = x^o

Also, we have been given that ∠ x = 84^o

Therefore, we have,

84 + x + x = 180^o

84 + 2x = 180^o

2x = 180 – 84

2x = 96

x = 48

Hence, the value of x = 48^o

Example 3 Find x° and y° from the given figure – 

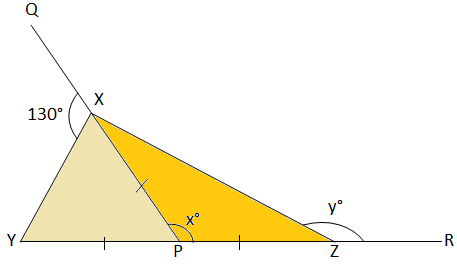
Solution We have been given a triangle XYP. Now in this triangle, we can see that a linear pair is formed by ∠YXP and ∠QXY. Since the sum of a linear pair is 180° therefore,

∠YXP + ∠QXY = 180°

⇒ ∠YXP = 180° – ∠QXY

  ⇒ ∠YXP = 180° – 130°

⟹ ∠YXP = 50°

Now, that we have found the value of ∠YXP, let us now find the value of ∠XPY. 

We have been given that XP = YP

Therefore, 

⟹ ∠YXP = ∠XYP = 50°.

Also, by the angle sum property of a triangle, we know that the sum of the three angles of a triangle is always equal to 180^o. Therefore,

∠YXP + ∠XYP + ∠XPY = 180°

⟹ 50° + 50° + ∠XPY = 180°

⟹ 100^o + ∠XPY = 180°

⟹ ∠XPY = 180° – 100^o

⟹ ∠XPY = 80°

Again, we can see that x° and ∠XPY form a linear pair. Therefore, we can say that,

x° + ∠XPY = 180°

⟹ x° + 80° = = 180°

⟹ x° = 180° – 80°

⟹ x° = 100°

Also, in ∆XPZ we have,

XP = ZP

Therefore, ∠PXZ = ∠XZP = z°

Therefore, in ∆XPZ we have,

∠XPZ + ∠PXZ + ∠XZP = 180°

⟹ x° + z° + z° = 180°

⟹ 100° + z° + z° = 180°

⟹ 100° + 2z° = 180°

⟹ 2z° = 180° – 100°

⟹ 2z° = 80°

⟹ z° = 80°280°2

⟹ z° = 40°

Therefore, y° = ∠XZR = 180° – ∠XZP

⟹ y° = 180° – 40°

⟹ y° = 140°

Example 4 Find the area of an isosceles triangle given its height as 8 cm and base as 6 cm?

Solution We have been given that the height of the triangle is 8 cm while the base of the triangle is 6 cm.

We are required to find the area of the isosceles triangle. We know that if we have the base and the height of a triangle, its area is given by 

Area of a Triangle = ½ × b × h

Therefore, substituting the values of the base and the height in the above formula, we have,

Area of a Triangle = ½ × 6 × 8 = 24

Hence, area of the given isosceles triangle = 24 sq. cm 

Key Facts and Summary

1. A triangle is said to be an Isosceles triangle if its two sides are equal. If two sides are equal, then the angles opposite to these sides are also equal.
2. A triangle is said to be a right isosceles triangle if apart from two sides being equal, one of the angles of the triangle is a right angle, i.e. 90^o_.
3. A triangle is said to be an acute isosceles triangle if apart from two sides being equal, all the three interior angles of the triangle are acute angles, i.e. all the three angles are less than 90^o.
4. A triangle is said to be an obtuse isosceles triangle if apart from two sides being equal, one of the angles of the triangle is an obtuse angle, i.e. greater than 90^o_.
5. The area occupied between these three sides is called the area of a triangle.
6. In general, the area of an Isosceles triangle is defined by ½ x b x h. This means that Area of a triangle = ½ x b x h, where b = base of the triangle (or any one side of the triangle), and h = Height of the triangle from that base (or side).
7. the area of an isosceles triangle if we know the two equal sides and the base is given by  ½ x Base x $\sqrt{(Equal\:Side)^2- \frac{1}{4}(Base)^2}$
8. Isosceles triangle theorem states that “In an isosceles triangle, the angles opposite to the equal sides are equal. Conversely, if the base angles of a triangle are equal, then the triangle is isosceles.”
9. The sum of the lengths of the sides of the isosceles triangle is called its perimeter.

Recommended Worksheets

Isosceles Triangles (Holiday Cruise Ship Themed) Math Worksheets
Obtuse Triangles (Toy Store Themed) Math Worksheets
Acute Triangles (City Themed) Math Worksheets