Home » Math Theory » Geometry » Rhombus

Rhombus

What is a Rhombus?

The rhombus is a parallelogram with four congruent sides (sides with equal lengths). A square is a special case of a rhombus. Therefore, a rhombus is a quadrilateral with four equal sides. Thus defining a rhombus we can say that:

Rhombus is a type of quadrilateral in which both the pairs of opposite sides are parallel and all the sides are having the same length and all the opposite angles are of equal measure it is also known as diamond any side of a rhombus can be chosen as the base of the Rhombus the perpendicular dropped on that side from the opposite vertex is known as its height or altitude

The properties of a rhombus are:

  1. A rhombus has two pairs of parallel sides
  2. The opposite angles in a rhombus are equal.
  3. A rhombus has four equal sides. This means that all its sides are equal.
  4. The diagonals of a rhombus bisect each other
  5. The diagonals of a rhombus are perpendicular each other

Let us understand each of the above properties through the below rhombus ABCD.

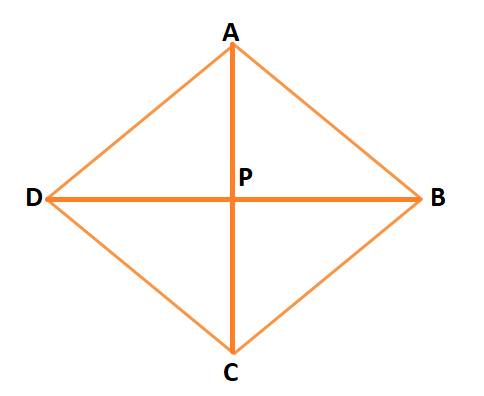
Properties of a Rhombus

Let us understand each of the above properties through the below rhombus ABCD.

  1. The rhombus ABCD has two pairs of parallel sides, namely ( AB and CD ) and ( AD and BC )
  2. The rhombus ABCD has all sides equal, i.e. AB = CD = AD = BC
  3. The rhombus ABCD has opposite angles equal, i.e. ∠A = ∠C and ∠B  = ∠D.
  4. The diagonals of the rhombus bisect each other, i,e. AP = PC and BP = PD 
  5. The diagonals of the rhombus ABCD are perpendicular to each other, i,e. AC ⟂ BD.

Area of a Rhombus

Rhombus is a parallelogram with all its sides equal. Some properties of a rhombus are:

  1. All sides of a rhombus are equal.
  2. Since all the sides of a rhombus are equal, it is also known as an equilateral quadrilateral.
  3. The diagonals of a rhombus bisect each other at right angles.
  4. Apart from using the sides, the area of a rhombus can also be calculated using its diagonals.

Area of a Rhombus using its Sides

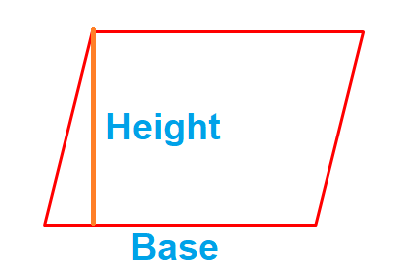
Since the rhombus is a parallelogram with all its sides equal, therefore, the formula for the area of a parallelogram also holds true for calculating the area of a rhombus.

Hence

Area of a Rhombus = Base x Height

Example:

Let us have a rhombus whose altitude is 7 cm and the rhombus has a perimeter of 180 cm.

Solution

We are given that

The altitude of the rhombus = 7 cm

The perimeter of the rhombus = 180 cm.

We need to find the area of the rhombus. In order to do so, we must first find the side of the rhombus.

Now, remember that the perimeter of a closed shape is the sum of all its sides. Since the rhombus is a quadrilateral with all its sides equal, we can say that if the rhombus has a side “a”, then

a + a +a + a =180 cm

4a = 180 cm

a = 45 cm

Hence the side (base) of the rhombus = 45 cm

Now, to calculate the area of a rhombus, we know that

Area of a Rhombus = Base x Height

Therefore,

Area = (45 x 7) cm2 = 315 cm2

Area of a Rhombus using its Diagonals

We can also calculate the area of a rhombus if we know its diagonals. The formula for calculating the area of the rhombus when we know its diagonals is given by:

Area of a Rhombus = $\frac{1}{2}$ x (Product of the diagonals)

If d1 and d2 are two diagonals of a rhombus, then,

Area of a Rhombus = $\frac{1}{2}$ x (d1 x d2)

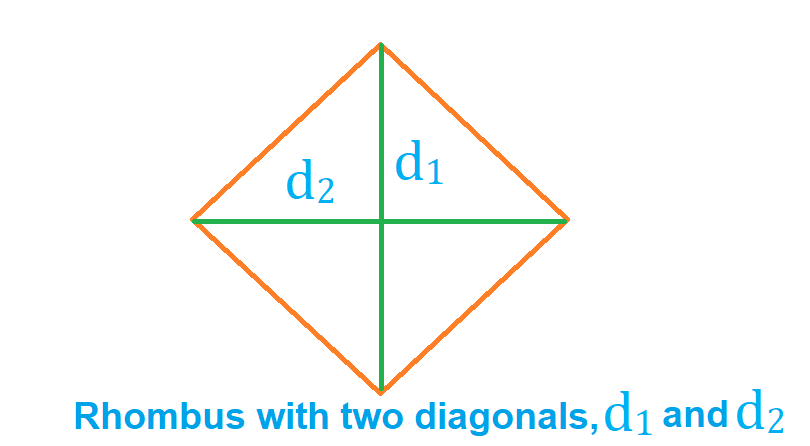
Example

If the two diagonals of a rhombus measure 9 cm and 12 cm respectively, find the area of the rhombus.

Solution

We know that we are given the diagonals, the area of the rhombus = $\frac{1}{2}$  x (d1 x d2)

Here, the two diagonals are 9 cm and 12cm.

So, let

D1 = 9 cm and d2 = 12 cm.

Area of the rhombus = $\frac{1}{2}$  x (d1 x d2) = $\frac{1}{2}$  x (9 x 12) cm2 = 54 cm2

Hence, area of the rhombus = 54 cm2

Perimeter of a Rhombus

The perimeter of a closed figure is given by the sum of all its sides. We know that rhombus is a type of quadrilateral that has all its sides equal. Therefore, the perimeter of a rhombus will be the total measure of its boundary. So, how do we find the perimeter of a rhombus? Let us find out.

Perimeter of a rhombus using sides

It is very simple to find the perimeter of a rhombus if we know the measure of its sides. To find the perimeter we need to find the sum of all its sides. Since a rhombus has 4 sides,

Hence

The perimeter of a rhombus = 4 x Side

Let us understand this by an example.

Suppose we have a rhombus whose each side is 4 cm. What would be its perimeter?

We have learnt that the perimeter of a rhombus is equal to the sum of its four sides. Also, in a rhombus all four sides are equal. The formula that we have for finding the perimeter of a rhombus is

Perimeter of a rhombus = 4 x Side

Here, side = 4 cm

Hence, perimeter of a rhombus = 4 x 4 = 16 cm

So, we now know how to find the perimeter of a rhombus using its sides. But what if instead of the sides, we know the diagonals of the rhombus? Can we still find its perimeter? Let us find out.

Perimeter of a rhombus using diagonals

We have learnt how to find out the perimeter of a rhombus when its sides are known. But when we know the diagonals instead of the sides, we use the Pythagoras theorem to find out the sides. Let us see how.

The following properties of a rhombus are used to find the value of the sides:

  • A rhombus is divided into 4 congruent right-angled triangles by its two diagonals.
  • The diagonals bisect each other at right angles.

Suppose we have the following rhombus, where the diagonal AC = a and the diagonal BD = b

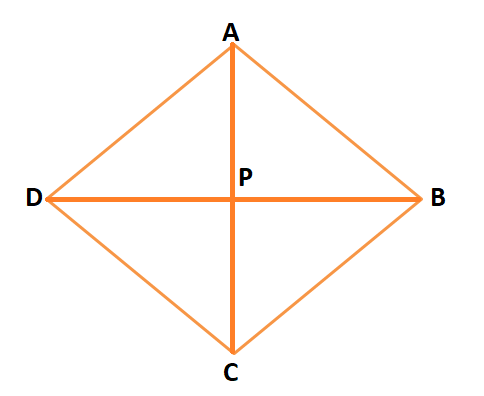
Now, let us consider the triangle APD. Using Pythagoras theorem we can say that,

AP2 + PB2 = AB2 ………………………………………………. (1)

Since the diagonals of a rhombus bisect at right angles, therefore, AP = PC =  =  

Simiallry, BP = PD = $\frac{BD}{2}=\frac{b}{2}$

Substituting the values of AP and AB is (1) we get

AB2 =  $\frac{a^{2}}{4}+\frac{b^{2}}{4}$

Therefore,

AB = $\frac{\sqrt{a^{2}+b^{2}}}{2}$ ……………………………….. (2)

Since we know that the Perimeter of the rhombus = 4a and AB is our side, we can substitute the value of AB in (2). We will get

Perimeter of a rhombus = $4\times \frac{\sqrt{a^{2}+b^{2}}}{2}=2\times\sqrt{a^{2}+b^{2}}$

Hence, the perimeter of a rhombus having diagonals a and b is given by $2\times\sqrt{a^{2}+b^{2}}$.

Let us understand this through an example

Suppose we want to find the perimeter of a rhombus having diagonals 6 cm and 8 cm respectively.

We know that

Perimeter of a rhombus having diagonals a and b is given by $2\times\sqrt{a^{2}+b^{2}}$.

We have been given the diagonals as 6 cm and 8 cm respectively. Let a = 6 cm and b = 8 cm

Substitute the values of a and b in the given formula we have,

Perimeter of a rhombus = $2\times\sqrt{a^{2}+b^{2}}$  

= $2\times\sqrt{6^{2}+8^{2}}$

= $2\times\sqrt{36+64}$

= $2\times\sqrt{100}$

 = $2 \times 10 = 20$ cm

Hence the perimeter of the given rhombus = 20 cm

Facts and Real Life Examples of a Rhombus

  1. The term ‘rhombus’ has been derived from the Greek word ‘rhombus (ῥόμβος)’ which means something that spins, and this term was eventually derived from the Greek verb ‘rhembō (ῥέμβω)’ which means to turn round and round.
  2. Rhombus can be found in a variety of things around us, such as a kite, windows of a car, rhombus-shaped earrings, the structure of a building, mirrors, and even a section of the baseball field.
  3. Squares are rhombuses. This is just a rhombus that has all right angles. While the most common term used to describe this shape in geometry is “rhombus” you may also hear the shape referred to as a rhomb or diamond. The plural for several of these is rhombuses or rhombi. 

Solved Examples

Example 1    

The side of a rhombus is 6.5 cm and its altitude is 4 cm. Find its area

Solution              

We have been given that the side of a rhombus is 6.5 cm and its altitude is 4 cm.

Now, we know that when we have been given a side of a rhombus and its height, then the area of a rhombus is calculated by the formula:

Area of a Rhombus = Base x Height

Substituting the given values in the above formula we get,

Area of a Rhombus = (6.5 x 4) cm2 = 26 cm2

Hence, area of the rhombus = 26 cm2

Example 2    

The area of a rhombus is 72 cm2. If its perimeter is 32 cm, find its altitude.

Solution              

We have been given that the area of a rhombus is 72 cm2 and its perimeter is 32 cm.

Now,

The perimeter of the rhombus = 32 cm.

This means that the sum of its four sides = 32 cm. Let one of the sides of the rhombus be ‘a’. Then

4a = 32 cm

⇒ a = $\frac{32}{4}$ = 8 cm

So, we now know the side of the rhombus as 8 cm.

Also, we have been given that area of a rhombus is 72 cm2           

We also know the formula for calculating the area of a rhombus which is

Area of a Rhombus = Base x Height

Substituting the values of the area and the side (base) in the above equation we have,

72 = 8 x Height

⇒ Height = $\frac{72}{8}$  cm = 9 cm

Hence the altitude (height) of the rhombus is 9 cm

Example 3    

If the side of a square is 4m and it is converted into a rhombus whose major diagonal is 6 m; find the other diagonal and the area of the rhombus.

Solution              

We have been given that the side of a square is converted into a rhombus. Let ABCD be the square such that it has been converted into a rhombus. We now have AB = 4 m. Also, we have been given that the length of the major diagonal is 6 m. Therefore,

Let AC be the major diagonal and BD be the minor diagonal of the rhombus. We have been given that,

AC = 6 m

We know that the diagonals of a rhombus bisect each other at right angles. Let the diagonals AC and BD bisect each other at right angles at O.

Therefore, we can say that,

OA = $\frac{1}{2}$ x AC = 3 m and ∠AOB = 90o

Using Pythagoras theorem, we will have

AB2 = OA2 + OB2

⇒ 42 = 32 + OB2

⇒ OB2 = 16 – 9

⇒ OB2 = 7

⇒ OB = $\sqrt{7}$ m

Now, we know that the diagonal BD = 2 x OB.

Therefore,

BD = 2 x $\sqrt{7}$ m =2$\sqrt{7}$ m

Hence the other diagonal of the rhombus = $2\sqrt{7}$ m

Now, we are required to find the area of the rhombus.

We know we can find the area of a rhombus if we know the values of its diagonals, the formula for which is

Area of a Rhombus = $\frac{1}{2}$ x (Product of the diagonals)

The values of the diagonal of the rhombus are 6 m and $2\sqrt{7}$ m respectively. Therefore,

Area of a Rhombus = $\frac{1}{2}\times (6\times 2\sqrt{7})$

Area of a Rhombus = $6\sqrt{7}$ m2

Hence, the area of the given rhombus = $6\sqrt{7}$ m2

Example 4    

One of the diagonals of a rhombus is equal to one of its sides. Find the angles of the rhombus.

Solution              

We have been given that, diagonals of a rhombus is equal to one of its sides. We need to find the angles of the rhombus.

Let ABCD be a rhombus such that its diagonals BD is equal to its sides.

This means that the sides AB = BC = CD = AD = BD

Also, we can say that

DABD and DBCD are equilateral. This means,

∠A = ∠C = 60° ……………………………….. (1)

Now, the angle formed at A and the angle formed at B are adjacent angles. Therefore, we can say that

∠A + ∠B = 180° ………………………………….. (2)

Substituting the value of ÐA from equation (1) in equation (2), we will get,

60° + ∠B = 180°

∠B = 180° – 60°

∠B = 120°

Now that we have the value of ∠B as well as ∠A, we can say that

Hence, ∠A = 60° = ∠C and ∠B = ∠D = 120°

Therefore, the four angles of the rhombus are ∠A = ∠C = 60° = and ∠B = ∠D = 120°

Example 5    

If the area of a rhombus is 48 sq. cm and one of its diagonals is 6 cm, then find its altitude

Solution          

Let ABCD be the given rhombus such that the diagonal AC = 6 cm.

Now, the area of rhombus ABCD = 48 sq. cm

Now, we know that

Area of a rhombus = $\frac{1}{2}$ x (Product of the diagonals)

⇒ Area of a rhombus = $\frac{1}{2}$ x 6 x BD

⇒ 48 = $\frac{1}{2}$ x 6 x BD

⇒ BD = 16 cm

Since the diagonals of a rhombus bisect each other at right angles, therefore,

OA = $\frac{1}{2}AC$ = 3 cm and OB = $\frac{1}{2}BD$ = 8 cm

Also, the triangle OAB is a right angle. Therefore, using Pythagoras theorem, we have,

AB2 = OA2 + OB2

⇒ AB2 = 32 + 82

⇒ AB2 = 9 + 64

⇒ AB2 = 73

⇒ AB = $\sqrt{73}$ cm

Now, the area of the rhombus ABCD = 48 cm2

This mean that Base x Altitude = 48 cm2

⇒ AB x Altitude = 48

⇒ Altitude x $\sqrt{73}$ = 48

⇒ Altitude = $\frac{48}{\sqrt{73}}$  cm

Hence, the altitude of the given rhombus = $\frac{48}{\sqrt{73}}$ cm

Remember

  1. A square, rectangle and rhombus are all parallelograms.
  2. A square is a rectangle and also a rhombus.
  3. A parallelogram is a trapezium and not a rhombus
  4. A kite is not a parallelogram
  5. A trapezium is not a parallelogram or a rhombus (as only one pair of opposite sides is parallel in a trapezium and we require both pairs to be parallel in a parallelogram.
  6. A rectangle or a rhombus is not a square
  7. A rhombus has two pairs of parallel sides
  8. The opposite angles in a rhombus are equal.
  9. A rhombus has four equal sides. This means that all its sides are equal.
  10. The diagonals of a rhombus bisect each other
  11. The diagonals of a rhombus are perpendicular each other
  12. Area of a Rhombus = Base x Height
  13. Area of a Rhombus = $\frac{1}{2}$ x (Product of the diagonals)
  14. Perimeter of a rhombus = 4 x Side
  15. Perimeter of a rhombus having diagonals a and b is given by $2\times\sqrt{a^{2}+b^{2}}$

Finding the Area of Triangle and Quadrilaterals 6th Grade Math Worksheets
Identifying Triangles, Quadrilaterals, Pentagons, Hexagons, and Cubes 2nd Grade Math Worksheets

Browse All Worksheets

Link/Reference Us

We spend a lot of time researching and compiling the information on this site. If you find this useful in your research, please use the tool below to properly link to or reference Helping with Math as the source. We appreciate your support!

  • "Rhombus". Helping with Math. Accessed on April 24, 2024. https://helpingwithmath.com/rhombus/.

  • "Rhombus". Helping with Math, https://helpingwithmath.com/rhombus/. Accessed 24 April, 2024.

  • Rhombus. Helping with Math. Retrieved from https://helpingwithmath.com/rhombus/.