What are Decimals?
The word decimal comes from the Latin word “Decem” which means 10. In algebra, a decimal number can be defined as a number whose complete part and the fractional part are separated by a decimal point.
Place Value System of Decimals
We know that each place in the place value table has a value ten times the value of the next place on its right. In other words, the value of a place is one-tenth of the value of the next place on its left. We observe that if one digit moves one place left to right its value becomes one-tenth ($\frac{1}{10}$ ) of its previous value and when it moves two places left to right its value comes one-hundredth ( $\frac{1}{100}$ ) of its previous term and so on. Therefore, if we wish to move beyond one’s place which is the case of decimals, we will have to extend the place value table by introducing the places of tenths ($\frac{1}{10}$ ), hundredths ($\frac{1}{100}$ ), thousandths ( $\frac{1}{1000}$ ) and so on.
Therefore, the place value table in case of a decimal number will be of the form –
| Thousands ( 1000 ) | Hundreds ( 100 ) | Tens ( 10 ) | Ones ( 1 ) | Tenths ($\frac{1}{10}$) | Hundredths ( $\frac{1}{100}$ ) | Thousandths ( $\frac{1}{1000}$ ) |
For example, the decimal number 257.32 in the place value system will be written as –
| Hundreds | Tens | Ones | Tenths | Hundredths |
| 2 | 5 | 7 | 3 | 2 |
This concept is important to learn as before addition or subtraction the decimal values will be required to be placed according to their place values.
Now, let us understand what we mean by like and unlike decimals
Like and Unlike Decimals
Consider the following group of decimals –
- 0.3, 0.7, 2.5, 12.7, 101.5
- 1.14, 22535, 0.6, 0.98
The number of digits on the right of the decimal place in a number is known as the number of decimal places.
The decimals having the same number of digits on the right of the decimals point are known as like decimals. For example, the first group of numbers above is a group of like decimals.
Similarly, the decimals having a different number of digits on the right of the decimals point are known as like decimals. . For example, the second group of numbers above is a group of unlike decimals.
Is it possible to convert, unlike decimals into like decimals? Let us find out.
Conversion of Unlike Decimals into Like Decimals
Let us consider two unlike decimals 3.5 and 4.75. It is important to note here that the first decimal number 3.5 has one digit after the decimal point while the second decimal number 4.75 has two digits after the decimal point.
To convert these unlike decimals into like decimals, we should have two decimal places in 3.5. We know that adding zero on the right of extreme right digits in the decimal part of a number does not alter its value.
Therefore, 3.5 can be written as 3.50
Now, 3.50 and 4.75 are like decimals.
Therefore, we can convert unlike decimals into like decimals by adding zeros to the right of the decimal point or by finding their equivalent decimal.
How are Decimal Numbers Represented on a Number Line?
Now, that we know what decimal numbers are and what is a number line, let us move to represent a decimal number on a number line. Representing decimal on a number line is defined as the plotting of decimal numbers on a number line.
An important aspect of decimals that needs to be understood for plotting them on a number line is that the decimals are placed between two integers.
Let us understand this by an example.
Let us take two numbers 0 and 1 draw a number line between them. We will have,
Next, let us split the space between 0 and 1 into tenths. We will have fractional values such as one-tenth, two-tenth, three-tenth and so on. It can be represented on the number line in the following manner.
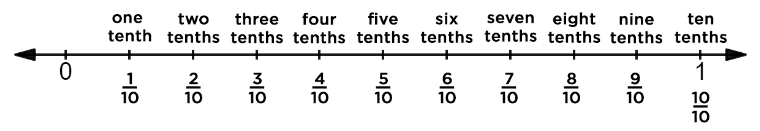
Now, we know that
One-tenth = 0.1
Two-tenth = 0.2
Three-tenth = 0.3 and so on.
Therefore, if we want to represent the above tenth values in the form of decimals we will have,

Further, if we wish to plot a decimal number say, 0.6, we will mark it as

In the above example, we had a simple decimal number and it was quite easy to plot it. But we know according to the place value system of decimals we have decimal numbers such as one-tenth ($\frac{1}{10}$), one-hundredth ( $\frac{1}{100}$ ), one-thousandth ( $\frac{1}{1000}$ ) etc. so how will we plot these numbers?
Representing Tenths on a Number Line
We already know how to represent fractions on a number line. Let us now represent tenths of a decimal on a number line. But, let us recall what we mean by tenths of a decimal?
Consider the following figure. It is divided into ten equal parts and one part is shaded. The shaded part represents one-tenth of the whole figure. It is written as $\frac{1}{10}$. $\frac{1}{10}$ is also written as 0.1 which is read as “ point one “ or “ decimal one “.
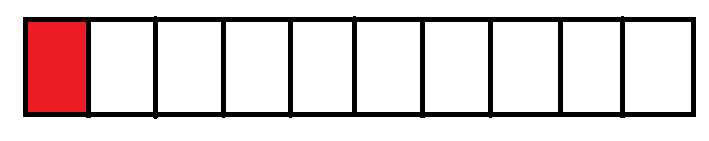
Thus the fraction $\frac{1}{10}$ is called one-tenth and is written as 0.1.
Now, let us represent tenths on a number line. We can understand this by an example.
Let us represent 0.4 on a number line. We can clearly see that there are 4 tenths in 0.4. Therefore in order to represent 0.4 on a number line, we will divide the unit length between 0 and 1 into 10 equal parts and take 4 parts as shown below –
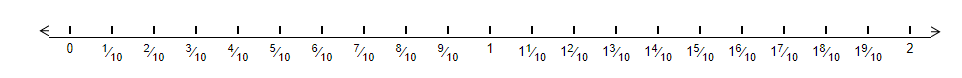
Now, we can know that 0.4 in fraction form is equal to 4/10. Hence we will mark 4/10 as 0.4 which is our desired mark on the number line.

Representing Hundredths on a Number Line
Let us now represent the hundredths of a decimal on a number line. But, let us recall what we mean by hundredths of a decimal?
If an object is divided into 100 equal parts, then each part is one-hundredth of the whole. This means that –
One thousandth = $\frac{1}{100}$ which in decimal form is equal to 0.01
If we take 7 parts out of 100 equal parts of an object, then 7 parts make $\frac{7}{100}$ of the whole and it is written as 0.07.
Now, let us represent hundredths on a number line. We can understand this by an example.
Let us represent the thousandth value of the number 7.45 on a number line.
To represent 7.4 on a number line, we first draw 10 lines dividing the total distance between 7 and 8 into 10 equal parts.

We can see that the arrow is four parts to the right of the whole number 7.
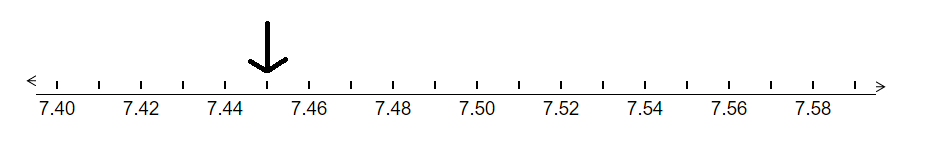
Similarly, to represent 7.45 on a number line, we first draw 10 lines dividing the total distance between 7.4 and 7.5 into 10 equal parts.
Representing Thousandths on a Number Line
Let us now represent the thousandths of a decimal on a number line. But, let us recall what we mean by thousandths of a decimal?
If an object is divided into 1000 equal parts, then each part is one-thousandth of the whole. This means that –
One thousandth = $\frac{1}{1000}$ which in decimal form is equal to 0.001
If we take 7 parts out of 1000 equal parts of an object, then 7 parts make $\frac{7}{1000}$ of the whole and it is written as 0.007.
Now, let us represent thousandths on a number line. We can understand this by an example.
Let us represent the thousandth value of the number 7.456 on a number line.
To represent 7.4 on a number line, we first draw 10 lines dividing the total distance between 7 and 8 into 10 equal parts.
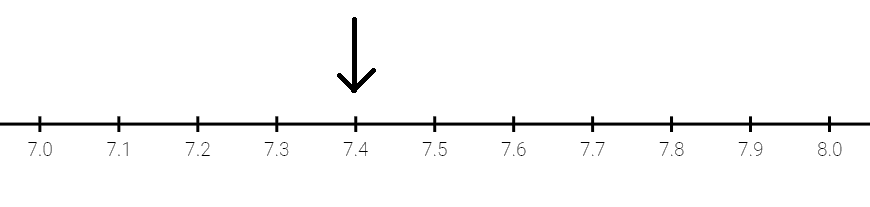
We can see that the arrow is four parts to the right of the whole number 7.
Similarly, to represent 7.45 on a number line, we first draw 10 lines dividing the total distance between 7.4 and 7.5 into 10 equal parts.
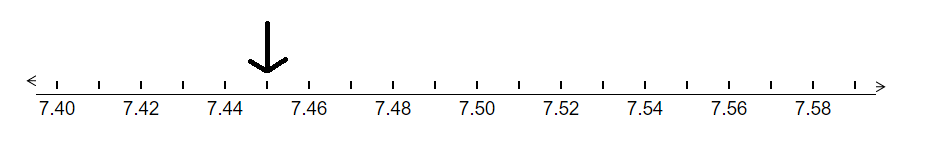
We can see that the arrow is five parts to the right of the decimal number 7.40.
Next, to represent 7.456 on a number line, we first draw 10 lines dividing the total distance between 7.45 and 7.46 into 10 equal parts.
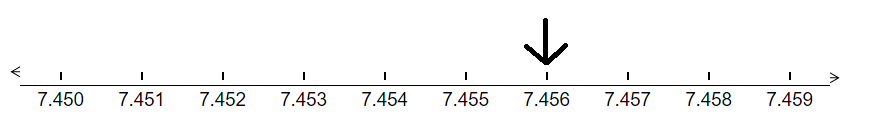
We can see that the arrow is six parts to the right of the decimal number 7.45
So, in this manner, we have represented the number 7.456 on the number line.
How to Compare Decimals?
In order to compare decimal numbers, the following steps will be followed –
- Obtain the decimal numbers.
- Next, we will compare the whole parts of the numbers. The number with the greater whole part will be greater. If the whole parts are equal we will move to the next step.
- Now, we will compare the extreme left digits of the decimal parts of the given decimal numbers. If the extreme left digits of the decimal part are equal, then compare the next digits and so on.
Let us understand it through an example.
Example
Suppose we wish to compare the decimal numbers 48.23 and 39.35
If we go by the above steps, we can clearly see that the given decimals have distinct whole number parts. This means that we can compare whole numbers parts only.
In the decimal number 48.23, the whole number part is 48
Similarly, in the decimal number 39.35, the whole number part is 39
Now, we know that
48 > 39
Therefore, 48.23 > 39.35
Let us take another example.
Example
Suppose we wish to compare the decimal numbers 0.58 and 0.84
If we go by the above steps, we can clearly see that the given decimals the whole number part in each fraction is equal to 0. This means that we will have to compare the decimal parts.
Now, we will check the extreme left digits in the decimal parts of 0.58 and 0.84 are 5 and 8 respectively.
Also, 5 < 8
Therefore,
0.58 < 0.84
Using Number Line to Compare Decimals
We can use the number line to compare decimals. Let us understand it through an example.
Example
Label the missing decimal numbers on the number line and compare them
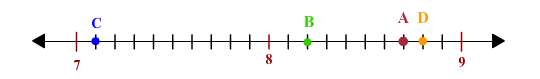
We have been given four numbers marked as A , B , C and D on a number line and we need to find out which decimal numbers they represent. Let us mark them one by one.
We will start with completing the marking of the lines that have not been marked on the given number line. It can be clearly seen that there are 10 lines between two whole numbers on the number line. This means that the lines represent one-tenth of the number in the decimal form. Therefore, the lines between 7 and 8 will be marked as 7 . 1 , 7 . 2 , 7 . 3 , 7 . 4 , 7 5 , 7 . 6 , 7 . 7 , 7 . 8 and d 7. 9. Similarly, We between the whole numbers 8 and 9 we have, 8 . 1 , 8 . 2 , 8 . 3 , 8 . 4 , 8 . 5 , 8 . 6 , 8 . 7 , 8 . 8 and 8 . 9. The number line so obtained will be –
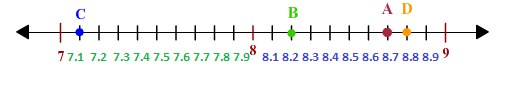
Now, we shall check the position of the four points on this number line.
We can see that from the number line above, the point A lies on the decimal number 8.7. Hence A = 8 . 7
Now, let us check the position of point B.
We can see that from the number line above, the point B lies on the decimal number 8.2. Hence B = 8 . 2
Now, let us check the position of point C.
We can see that from the number line above, the point C lies on the decimal number 7 . 1. Hence C = 7 . 1
Now, let us check the position of point D.
We can see that from the number line above, the point D lies on the decimal number 7 . 8 . Hence D = 7 . 8
Therefore, we have,
A = 8 . 7
B = 8 . 2
C = 7 . 1
D = 8 . 8
Now, we know that the numbers on a number line increase from left to right. Therefore, we will have,
7.1 < 8.2 < 8.7 < 8.8
How to place decimals in order?
Now that we have learnt how to compare decimals let us learn how to put them in order. by saying how to put them in order, it is meant that putting them in ascending order or in descending order. Recall that ascending order means putting the numbers from smallest to largest order and descending order means placing the numbers from largest to smallest order. Let us see how to place decimal numbers in order.
We can use the following steps to place the decimal numbers in order –
- In the first step, we set up a table with the decimal point in the same place for each number.
- Now, we fill in the empty spaces with zeroes.
- Next, we compare the decimal numbers using the first column on the left.
- If the digits are equal we move to the next column on the right.
- To place the decimals in ascending order, we choose the smallest number first while if we wish to place the decimals in descending order, we choose the largest number.
Let us understand it through an example.
Example
Suppose we want to place the following decimals in ascending order –
1.307, 1.36 and 0.7
Let us perform the above steps for this purpose –
In the first step, we set up a table with the decimal point in the same place for each number. So we will have,
| Ones | Decimal Point | Tenths | Hundredths | Thousandths |
| 1 | . | 3 | 0 | 7 |
| 1 | . | 3 | 6 | |
| 0 | . | 7 |
Now, we fill in the empty spaces with zeroes. We will have
| Ones | Decimal Point | Tenths | Hundredths | Thousandths |
| 1 | . | 3 | 0 | 7 |
| 1 | . | 3 | 6 | 0 |
| 0 | . | 7 | 0 | 0 |
Next, we compare the decimal numbers using the first column on the left. We can see that two of them are “1”s and the other is a “0”. Ascending order needs smallest first, so we will choose “0” first. So we get the first number 0.7 and we will remove it from the list.
Now, we will compare the tenths. We can see that there are two values for the tenth value of “3” So, we shall move to the hundredth value. We can see that we have “0” and “6” in the hundredth value. Now, 0 < 6, therefore,
1.30 < 1.36
So the next numbers in order will be 1.30, 1.36.
Hence, the ascending order of decimals will be 0.7 < 1.30 < 1.36
Key Facts and Summary
- A decimal number can be defined as a number whose complete part and the fractional part are separated by a decimal point.
- The Place Value System is the system in which the position of a digit in a number determines its value. The place value of a digit in a number is the value it holds to be at the place in the number.
- The decimals having the same number of digits on the right of the decimals point are known as like decimals.
- The decimals having a different number of digits on the right of the decimals point are known as like decimals.
- We can convert unlike decimals into like decimals by adding zeros to the right of the decimal point or by finding their equivalent decimal.
Recommended Worksheets
Ordering Decimals (Holi Themed) Math Worksheets
Basic Operations of Decimals (Hanukkah Themed) Math Worksheets
Word Problems Involving Decimals (Market Themed) Math Worksheets









