Formulas

Introduction

Formulas are like magical spells in the world of mathematics. Just as spells help wizards and witches perform wonders, formulas help us find answers to various mathematical problems. But unlike spells, formulas are not mysterious or secretive but based on logic and reasoning. This article focuses on some basic algebraic formulas and basic formulas in Geometry.

Grade Appropriateness

This article suits students in grades 3 through 9, ages 8 to 15.

Math Domain

Formulas are found across all math domains, but this article will delve into basic algebra and geometry.

Applicable Common Core Standards

CCSS.Math.Content.6.EE.B.6: Use variables to represent numbers and write expressions when solving a real-world or mathematical problem.

CCSS.Math.Content.7.EE.B.3: Solve real-life and mathematical problems using numerical and algebraic expressions.

Definition of the Topic

A formula is a concise approach to symbolically express information. In math, formulas represent relationships between quantities and calculate unknown values.

Key Concepts

Variables: Symbols (often letters) that represent unknown numbers.

Equation: A statement with a “=” symbol indicating two expressions are equal, representing the same number.

Solving: The process of finding the value of a variable.

Discussion with Illustrative Examples

A formula is a mathematically based fact or rule. A formula has an equal sign (=) and two or more variables (x, y, etc.) with an unknown and solvable value.

Formulas are helpful because once you know the value of the required quantities, you can use the formula to determine the missing value.

Algebraic Formulas

Algebraic formulas lay the groundwork for many mathematical concepts, including equations, polynomials, trigonometry, etc. Here are some of the algebraic formulas that are used the most.

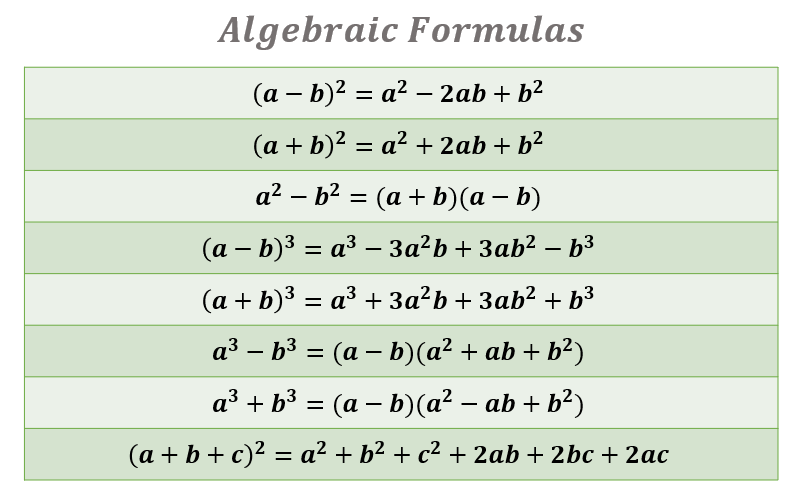
Geometric Shapes Formulas

Formulas are used in geometry to determine the dimensions, perimeter, area, surface area, volume, etc., of various shapes. The flat, two-dimensional (2D) shapes only have length and width, while three-dimensional (3D) shapes are shapes in space where they have length, width, and height.

Examples with Solution

Example 1

Find the area of the square.

Solution

The formula of a square is calculated using the formula Area=(side)². Hence the area of the square is (2x+y)². 

To expand the given polynomial, use the algebraic formula (a+b)²=a²+2ab+b² where a=2x and b=y. Hence, we have,

(2x+y)² = (2x)²+2(2x)(y)+y²

(2x+y)² = 4x²+4xy+y²

Therefore, the square has an area of (2x+y)² OR 4x²+4xy+y²

Example 2

What is the area of a square with a perimeter of 48 cm?

Solution

Since the perimeter of the square is 48 cm, the length of each side must be 48÷4=12 cm.

Therefore, the area of a square =(12 cm)²=144 cm².

Example 3

Find the value of 13²-5².

Solution

Let us calculate the value of 13²-5² using the algebraic formula

a²-b² = (a+b)(a-b). So, we have,

13²-5² = (13+5)(13-5)

13²-5² = (18)(8)

13²-5² = 144

Example 4

A rectangular game room has a perimeter of 76 cm. The length is 2 cm, more than twice the width. What are the dimensions of the room?

Solution

Let x be the width of the room and the length be 2x+2.

Since the room is rectangular, we shall use the formula 2l+2w=Perimeter_Rectangle.

Substituting the values into the equation and calculating x, we have,

2(2x+2)+2x=76

4x+4+2x=76

6x+4=76

6x=76-4

6x=72

x=$\frac{72}{6}$

x=12 cm  (width of the room)

For the length of the room, substitute x=12 cm into 2x+2.

2(12)+2 = 26 cm (length of the room)

Therefore, the dimensions are width=12 cm and length=26 cm.

To check, we may substitute the calculated dimensions into the formula.

Perimeter_Rectangle = 2(26) + 2(12)

Perimeter_Rectangle = 52+24

Perimeter_Rectangle = 76 cm.

Real-life Application with Solution

Math formulas have countless practical applications, especially in our day-to-day lives. Formulas are used to solve problems correctly in more complex ideas like algebra and geometry. 

Problem 1

Suppose your school is hosting a fundraiser. Each student contributes \$3, and the school contributes an initial \$50. How much will there be if 20 students contribute?

Solution

The formula that we will use would be:

Total=50+3s

Where “s” represents the number of students.

Substituting 20 into the equation, we have,

Total=50+3(20)

Total=50+60

Total=110

The fundraiser would have $110 if 20 students contributed.

Problem 2

Suppose you have some money saved up and decide to save a fixed amount weekly. If you saved for four weeks, how much would you have?

Solution

To represent your total savings, use a formula!

If you have \$10 and save \$5 every week, after “w” weeks, your savings would be:

Savings=10+5w

Here, “w” is the variable representing the number of weeks.

Using our formula: 

Savings=10+5(4)

Savings=10+20

Savings=30

You would have $30 after four weeks!

Practice Test

1. If you had \$15 and saved \$6 every week, how much would you have after five weeks?

2. Using the formula Area=length×width, what is the area of a rectangle that is 10 units long and 5 units wide?

3. If each book costs \$7 and you bought “b” books, how much would you spend in total?

4. A car travels 60 miles every hour. Using the formula Distance=speed×time, how far would the car travel in 3 hours?

5. A movie ticket costs \$9. If “t” friends are going, how much would all the tickets cost?

Answers:

1. Formula: Total=15+6w, where w is the number of weeks

Total=15+6(5)

Total=15+30

Total=45

You would have \$45 after five weeks.

2. Area=length×width

Area=10×5

Area=50

3. Formula: Total=7b, where “b” is the number of books

4. Distance=speed×time

Distance=60 miles per hour ×3 hours

Distance=180 miles

5. Formula: Total=9t, where “t” is the number of friends going to the movie

Frequently Asked Questions (FAQs)

Why do we use formulas?

Formulas help simplify complex problems and make calculations systematic and consistent.

Where else are formulas used?

They are everywhere! Formulas are used in science, engineering, finance, and even in everyday life, like cooking or carpentry.

Is it essential to memorize all formulas?

Not always. It is more important to understand how formulas work. With practice, you will remember the ones you use frequently.

What if I use the wrong formula?

Mathematics encourages trial and error. If one approach does not work, you can always try another.

Can I create my own formulas?

Absolutely! If you identify a pattern or relationship, you can develop a formula for it. That is how many formulas were created!

We hope this journey into the world of formulas has been enlightening. Remember, formulas are tools to help solve problems, and with practice, you’ll become a formula wizard in no time! Happy calculating!

Recommended Worksheets

Formulas (National Aviation Day Themed) Math Worksheets
Equation of a Straight Line (National Maritime Day Themed) Math Worksheets
Problem Solving – Addition and Subtraction (World Teachers’ Day Themed) Math Worksheets