Long Multiplication
We know that multiplication is a form of repeated addition. While it is easy to multiply two numbers that are of one-digit or two digits using multiplication tables, the same does not hold true for larger numbers. Multiplication of large numbers, where either the multiplicand is a two or more digit number or the multiplier is a two or more digit number involves a different method of multiplication known as Long multiplication.
Remember, the way we multiply the numbers remains the same, this method is only a way to arrange the numbers in such a manner so as to make the process of multiplication easier and faster.
In this method, we split the numbers into columns and multiply the numbers by the multiplicand one by one. There are two scenarios when using this method.
Let us understand them one by one
Long Multiplication without Regrouping
This method comes into force when we have numbers that do not involve carrying forward any numbers to the digit at the next place value. Let us understand it through an example.
For example, multiply 1021 by 3
Solution:
We will use the following steps to obtain our result.
Step 1: First we write the multiplicand and the multiplier in columns.
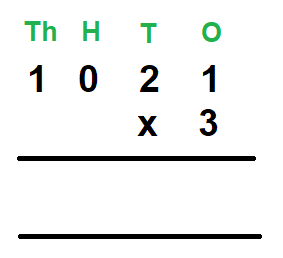
Step 2: Now, we multiply the number at the one’s place by 3. We get
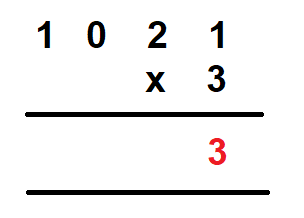
Step 3: Now, we multiply the number at the ten’s place by 3. We get

Step 4: Next, we multiply the number at the hundred’s place by 3. We get
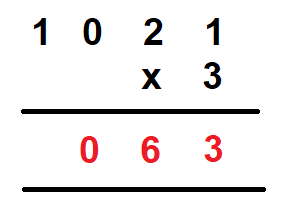
Step 5: Last, we multiply the number at the thousand’s place by 3. We get
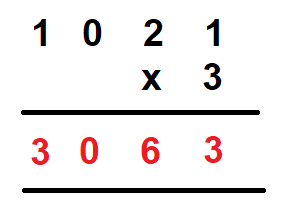
Therefore, 1021 x 3 = 3063
Long Multiplication with Regrouping
In the above case, we have small multiplications that did not involve two-digit results at any step. But in the case of larger numbers, there will be a need to carry forward the number to the number at the next place value. This is called Multiplication with Regrouping. Let us understand it through an example.
For example, Multiply 5092 by 5
Solution
We will use the following steps to obtain our result.
Step 1: First we write the multiplicand and the multiplier in columns.
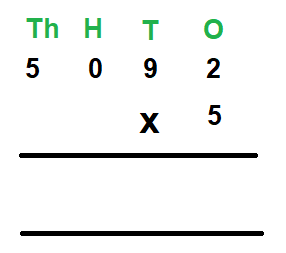
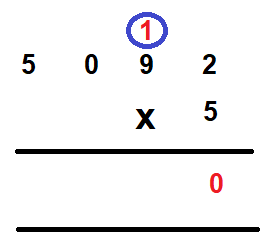
Step 2: Multiply the one’s digit by 5. We have 2 x 5 = 10. Write 0 in the one’s column and carry over 1 to the ten’s column.
Step 3: Multiply ten’s digit by 5. We get 9 x 5 = 45. Add 1 that was carried over to it to get 45 + 1 = 46. Now, write 6 in the ten’s column and carry over 4 to the hundred’s column.
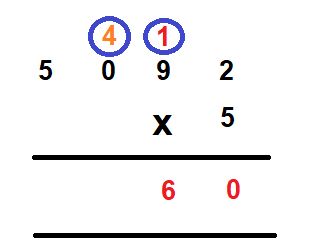
Step 4: Multiply the hundred’s digit by 5. We get 0 x 5 = 5. Now add 4 that was carried over to it and we get 4. Write 4 in the hundred’s column.
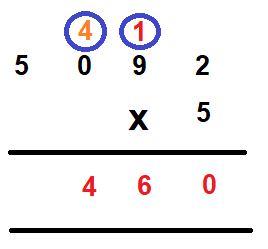
Step 5: Multiply 5 with thousand’s digit. We get 5 x = 25. Write 5 in the thousand’s column and 2 in the ten thousand’s columns.
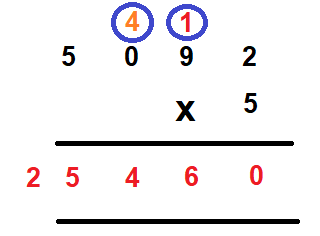
Hence, the final product is 25460.
Long Multiplication when the multiplier is a two digit number
The method for Long Multiplication when the multiplier is a two digit number i.e when the multiplier is greater than 9, involves the following steps –
- First, we write the multiplicand and the multiplier in columns.
- First, multiply the number at the one’s place of the multiplier with all the numbers of the multiplicand and write them horizontally.
- Make sure you write numbers from right to left and each number below the corresponding place value of the multiplicand.
- Now, move to the next line.
- Place a 0 at the one’s place of this line.
- Now, look for the digit at the ten’s place of the multiplier. Multiply the number at the ten’s place of the multiplier with all the numbers of the multiplicand and write them horizontally in the line where you had marked 0.
- Since the multiplier was a two-digit number, you have now reached the end of the multiplier.
- Add the numbers vertically according to their place values.
- The number so obtained is your result.
Let us understand this by an example
For example, multiply 132 by 13
Solution
1. First, we write the multiplicand and the multiplier in columns.
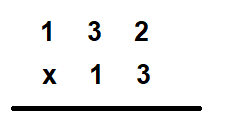
2. First multiply the number at the one’s place of the multiplier with all the numbers of the multiplicand and write them horizontally.
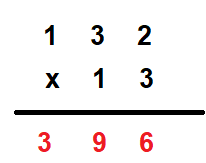
3. Place a 0 at the one’s place of the next line

4. Now, look for the digit at the ten’s place of the multiplier. Multiply the number at the ten’s place of the multiplier with all the numbers of the multiplicand and write them horizontally in the line where you had marked 0.
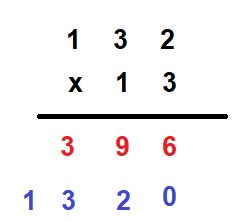
5. There is no more number in the multiplicand. Now, add the numbers vertically according to their place values.

6. The final answer is 1716. Hence 132 x 13 = 1716
Long Multiplication when the multiplier is a three digit number
The method for Long Multiplication when the multiplier is a three digit number, i.e. when the multiplier is greater than 99, involves the following steps:
- First, we write the multiplicand and the multiplier in columns.
- First, multiply the number at the one’s place of the multiplier with all the numbers of the multiplicand and write them horizontally.
- Make sure you write numbers from right to left and each number below the corresponding place value of the multiplicand.
- Now, move to the next line.
- Place a 0 at the one’s place of this line.
- Now, look for the digit at the ten’s place of the multiplier. Multiply the number at the ten’s place of the multiplier with all the numbers of the multiplicand and write them horizontally in the line where you had marked 0.
- Again move to the next line.
- Place a 0 at the one’s as well as ten’s place of this line.
- Now, look for the digit at the hundred’s place of the multiplier. Multiply the number at the hundred’s place of the multiplier with all the numbers of the multiplicand and write them horizontally in the line where you had marked the two zeros.
- Since the multiplier is a three-digit number, you have reached the end of the multiplier
- Add the numbers vertically according to their place values.
- The number so obtained is your result.
For example, multiply 364 by 123
Solution
1. First we write the multiplicand and the multiplier in columns
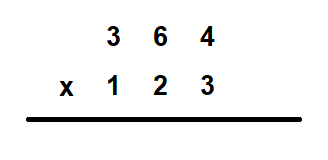
2. Now multiply the number at the one’s place of the multiplier with all the numbers of the multiplicand and write them horizontally.
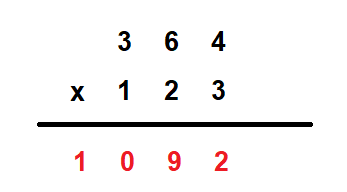
3. Place a 0 at the one’s place of the next line
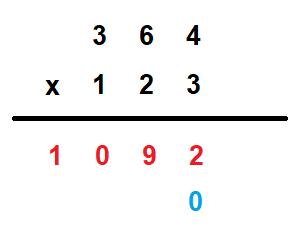
4. Now, look for the digit at the ten’s place of the multiplier. Multiply the number at the ten’s place of the multiplier with all the numbers of the multiplicand and write them horizontally in the line where you had marked 0.

5. Place a 0 at the one’s as well as ten’s place of the next line.
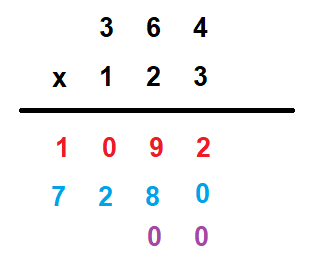
6. Now, look for the digit at the hundred’s place of the multiplier. Multiply the number at the hundred’s place of the multiplier with all the numbers of the multiplicand and write them horizontally in the line where you had marked the two zeros.
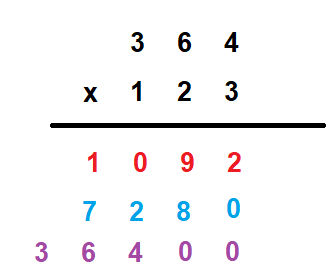
7. There is no more number in the multiplicand. Now, add the numbers vertically according to their place values.
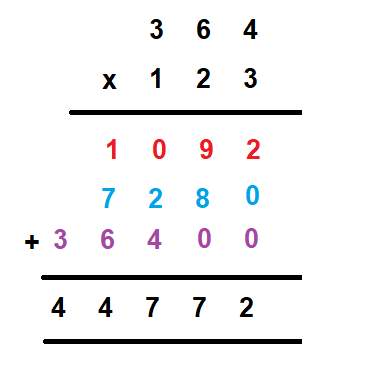
8. Hence the final product is 44,772. We can say that 364 x 123 = 44772
General Steps for Long Multiplication
Let us now summarise the steps involved in Long Multiplication irrespective of the number of digits of the multiplier. The steps are:
- First, we write the multiplicand and the multiplier in columns.
- First, multiply the number at the one’s place of the multiplier with all the numbers of the multiplicand and write them horizontally.
- Make sure you write numbers from right to left and each number below the corresponding place value of the multiplicand.
- Now, move to the next line.
- Place a 0 at the one’s place of this line.
- Now, look for the digit at the ten’s place of the multiplier. Multiply the number at the ten’s place of the multiplier with all the numbers of the multiplicand and write them horizontally in the line where you had marked 0.
- Again move to the next line.
- Place a 0 at the one’s as well as ten’s place of this line.
- Now, look for the digit at the hundred’s place of the multiplier. Multiply the number at the hundred’s place of the multiplier with all the numbers of the multiplicand and write them horizontally in the line where you had marked the two zeros.
- Continue in this manner by adding an extra zero in each line until you have reached the end of the multiplier
- Add the numbers vertically according to their place values.
- The number so obtained is your result.
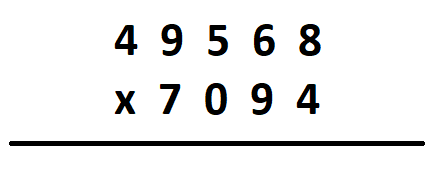
For example, multiply 49568 by 7094
Solution
1. First, we write the multiplicand and the multiplier in columns
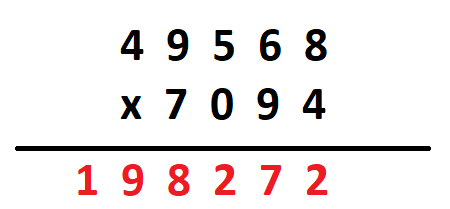
2. Now multiply the number at the one’s place of the multiplier with all the numbers of the multiplicand and write them horizontally.
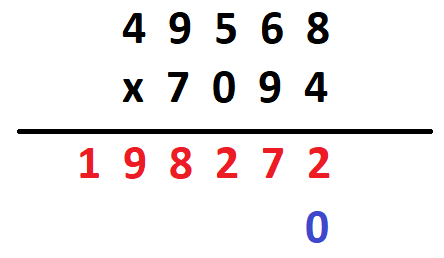
3. Place a 0 at the one’s place of the next line
4. Now, look for the digit at the ten’s place of the multiplier. Multiply the number at the ten’s place of the multiplier with all the numbers of the multiplicand and write them horizontally in the line where you had marked 0.
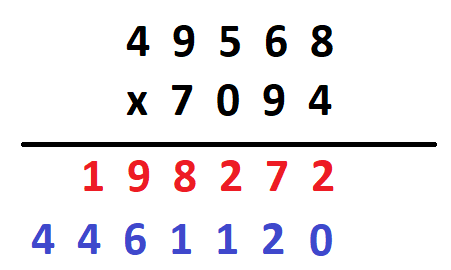
5. Again move to the next line. Place a 0 at the one’s as well as ten’s place of this line.
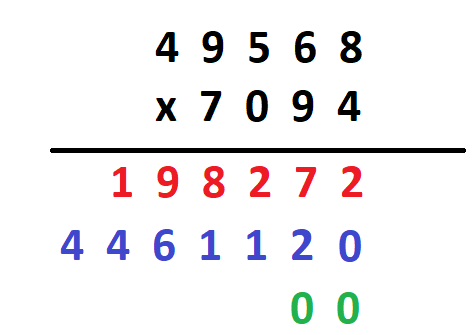
6. Now, look for the digit at the hundred’s place of the multiplier. Multiply the number at the hundred’s place of the multiplier with all the numbers of the multiplicand and write them horizontally in the line where you had marked the two zeros.
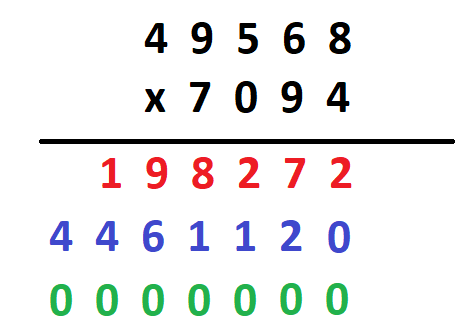
7. Again move to the next line. Place a 0 at the one’s, ten’s and hundred’s place of this line.
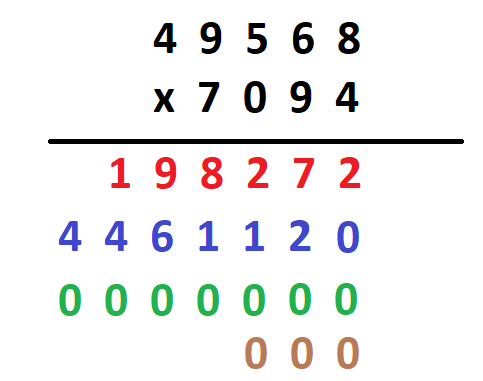
8. Now, look for the digit at the thousand’s place of the multiplier. Multiply the number at the thousand’s place of the multiplier with all the numbers of the multiplicand and write them horizontally in the line where you had marked the three zeros.
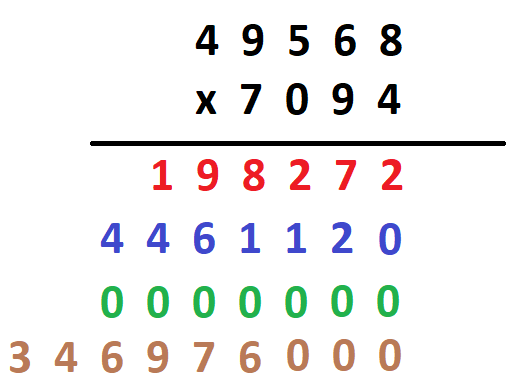
9. Since the multiplier is a four-digit number, you have reached the end of the multiplier. Add the numbers vertically according to their place values.
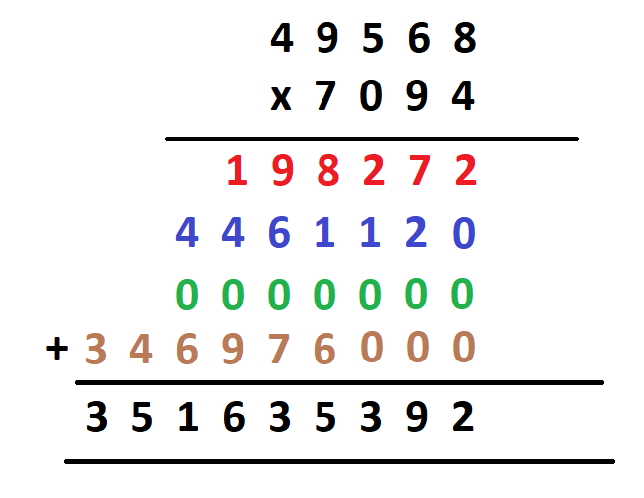
10. The number so obtained is your result. Hence 49568 x 7094 = 351635392
Multiplication by 10, 100 and 1000
- To multiply a number by 10, put 0 to the right of the number. For example, 435 x 10 = 4350
- To multiply a number by 100, put two zeros to the right of the number. For example, 435 x 100 = 43500
- To multiply a number by 1000, put three zeros to the right of the number. For example, 435 x 1000 = 435000
- To multiply a number by the product of 10, 100 and 1000 with the counting numbers, multiply the numbers with the non-zero numbers and then add the number of zeros to the result. For example, multiply 45 by 200. To solve this, we will first multiply 45 by 200. We get 45 x 2 = 90. Now we add 2 zeros (as 200 had 2 zeros) to the right of 90. We get 9000.
- Hence, 45 x 20 = 9000
Techniques for Faster Long Multiplication
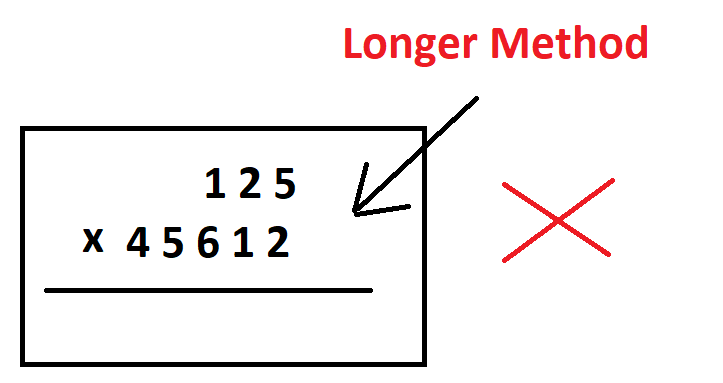
1. Multiplication is communicative as well as associative. So, it does not matter out of the two numbers, which one you mark as a multiplier and which number as the multiplicand. Therefore, it is always good to use the shorter number as a multiplier as it will ease your calculation. For example, if we want to multiply 125 by 45612, note that we should use 125 as a multiplier instead of 45612 as it is a shorter number. Therefore,
2. Out of the two numbers that are to be multiplied, check which number has the maximum number of zeros. The number that has more number of zeros should be used as the multiplier.

Some more Examples
A travel agency charges £80563 for a 7-day trip to Europe per passenger. If 8790 passengers take a trip in a year, how much has the travel agency earned during that year?
Solution: We have been given that the travel agency charges £80563 for a 7-day trip to Europe per passenger.
So, cost of one passenger for the 7-day trip = £80563
Total number of passengers in a year = 8790
We are required to find out the earnings of the travel agency in that year. To find this, we will need to multiply £80563 by 8790.
(Note: We have chosen the smaller number as the multiplier)
So, using the steps explained above for long multiplication, we get
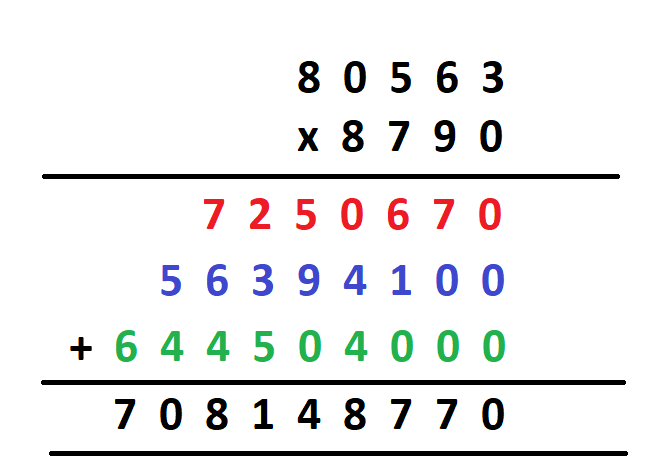
Hence, the travel agency earns £70,81,48,770 in that year.
In a factory, 144 cricket balls can be packed in one carton. There are 675 such cartons in the factory. How many balls can be packed in these cartons?
Solution: We have been given that –
Number of cricket balls that can be packed in one carton = 144
Number of cartons = 675
We need to find out many balls can be packed in 675 cartons. In order to find this, we need to multiply 675 by 144
(Note: We have chosen the smaller number as the multiplier)
So, using the steps explained above for long multiplication, we get

In a town there are 146 rows of houses. If there are 25 houses in each row, how many houses are there in the town?
Solution: We have been given that there are 146 rows of houses in a town.
The number of houses in each row is 25.
We need to find the total number of houses in the town. In order to find this, we will have to multiply 146 by 25.
Note: We will take 25 as the multiplier for two reasons; first it is a multiple of 5 which makes multiplication easier and second, it is a smaller number than 146.
So, upon multiplying 146 by 25 we get –
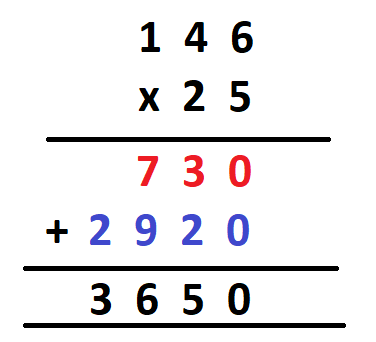
Hence, the total number of houses in the town is 3650
Maria has a bakery that can produce 2435 large packets of cookies in a month. Each packet of cookies contains 35 cookies. How many cookies are produced in the bakery in a month?
Solution: We have been given that –
Number of large packets of cookies that can be produced in a month at Maria’s Bakery – 2435
Number of cookies in each packet = 35
We are required to find the total number of cookies that can be produced in Maria’s bakery in a month. To find this, we will need to multiply 2435 by 35.
Note: We will take 35 as the multiplier for two reasons; first it is a multiple of 5 which makes multiplication easier and second, it is a smaller number than 2435.
So, upon multiplying 2435 by 35 we get –
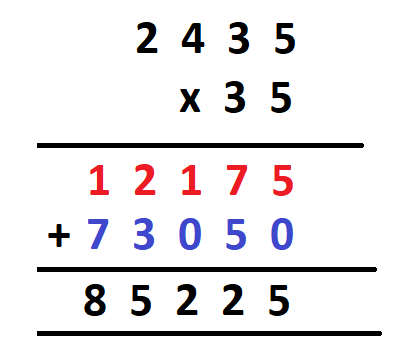
Hence, the total number of cookies that can be produced in Maria’s bakery in a month = 85225
Recommended Worksheets
Multiplicative Comparisons and Equations 4th Grade Math Worksheets
Multiplying and Dividing Multi-Digit Whole Numbers 5th Grade Math Worksheets
Order of Operations (PEMDAS) (Work from Home Themed) Worksheets









