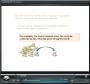
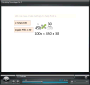
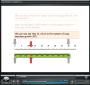
This section includes guidance on how to calculate percentage values. Note: Be sure to discuss real-life uses of percentages with your child. Good examples include percentage used for increases in pay/ wages, for interest from savings at a bank, and for results in exams at school.
There are several different types of task involving percentages. In addition to converting between fractions, decimals, and percent, there are problems requiring any one of three unknowns to be found as the examples below show:
- Find the percentage of a quantity e.g. What is 35% of 180?
- Expressing a quantity as a percent of another. e.g. 12 is what percentage of 18?
- Finding the whole. e.g. 35 is 70% of a number. What is the number?
The mini-lessons below will show how these types of problems can be solved.
Percentage Mini-lessons
The three short mini-lessons above are on calculating with percent. Lesson #1 is an introduction to percent, lesson #2 shows how to calculate a percentage e.g. what is 20% of 70? Lesson #3 shows both how to find a percent e.g. What percent is 25 out of 40? and also how to calculate the base e.g. If 30% of an amount is 45, what is the amount?
What else is in the percentages section?
 | You’ll find a number of printable percentage worksheets for practicing with percentages, |
| As mentioned above, there is illustrated, step-by-step instruction on how to calculate percentages. |  |
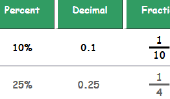 | There are examples showing how to convert between percents and decimals and fractions. |
 | An online percentage calculator is also provided and it’s an excellent way to check your answers. |
You might also like to try this “How much is shaded?” target game for a bit of practice with percent.
Choose the resources you need from either the links above or from the top menu options.
Keep the points below in mind when you are tutoring your children on percent. Percent is a very commonly used mathematical concept in everyday life and it has also proven to be a hard concept for many to grasp and understand.
Teaching Percent
Instruction should focus on what percent is as opposed to just looking at procedures and the quickest way to compute it. Similarly, there should be a move away from just imparting the knowledge that 50% is one-half. The importance of the base of 100 should be introduced early and often.
The language of percent is extremely important. Students need to understand proportion and must be able to express this understanding using the language of percent. They must identify implicit referents. e.g. before solving a problem they must ask themselves, “30% of what?”
Lots of graphical representations should be used as examples to help develop students’ proportional reasoning skills. These should use real-life examples whenever possible that are relevant to students. e.g. sports performance as opposed to interest rates.
Typical errors and their causes
The language surrounding percent can often be difficult for students. It often needs to be very precise. A very small change in wording can be associated with a completely different type of situation and problem. Questions are often not explicitly complete and students have to infer what the question refers to. e.g. “The unemployment rate is 9% .” not “The unemployment rate is 9% of the working-aged population.”
The issues above cause students to make errors such as those below:
- Ignoring the % symbol and then adding it back into the solution arbitrarily
- Incorrectly applying conversion rules e.g. since 45% = 0.45, then 7% = .7 or 140% = .14
- A general tendency to manipulate numerals rather than to apply reasoned thinking
- Seeing the % symbol as a label in the same way as units are e.g. 6m for 6 meters