Prime and Composite Numbers

Prime and Composite Numbers

The two sorts of numbers are prime and composite numbers, which differ in the amount of elements they contain. A prime number is one with only two elements, whereas a composite number has more than two. A factor is a value that has the ability to equally divide a number or an expression. The properties of prime numbers and composite numbers, as well as their differences, examples, and tables, are presented in this article. This article’s chart of prime and composite numbers can assist you in quickly identifying them.

First of all we define prime and composite numbers then make a difference between them.

Prime numbers

Prime numbers are those with only two elements, namely 1, and the digit itself. Think about the number 5, which really only contains two factors: 1 and 5. This indicates that the integer is prime. List the number 6, for example, which has far more that two factors, namely 1, 2, 3, and 6. As a result, 6 isn’t a prime number. Now, if we consider the number 1, we can see that it only has one factor. As a result, it isn’t a prime number, because a prime number must have precisely two elements. This means that 1 is a unique number, as was neither a prime nor even a composite number.

Hence, A prime number is a number higher than 1 that has exactly two elements, namely 1 and the number itself. In other words, a prime number is something that can be split into equal groups. Only if a number can be factorized as a product of two numbers can it be split into groups with equal amounts of objects. For example, 7 cannot be divided into equal-sized groups. This really is due to the fact that 7 can only be factorized in the following way:

11 × 1 = 11

1 × 11 =  11

This implies that the only elements of 7 are 1 and 7. Because it cannot be split into groups of equal numbers, 7 is a prime number. Definition: – A prime number is any whole number bigger than one that is split exclusively by one and itself.

Examples

17 = 1 × 17 so, it has two factors 1 and 17 itself. So, it is prime number

Further examples of prime numbers are as follows

3 , 7 , 13 , 19 , 23 , 29,  31 , etc

The study underlying number theory requires primes. Almost all scientific and numeric theorems use prime numbers or can be traced back to them in some way. Prime numbers are also significant in cryptography research. The Cryptography algorithm is based on the factorization of an integer into primes. Lastly, prime numbers are used in almost every branch of mathematics. Prime numbers are the “basic components” of numbers, and understanding them is essential for understanding how figures are linked to one another.

Prime Numbers and Their Aspects

The following are some of the most essential qualities of prime numbers:

• A prime number is a whole number that is greater than or equal to one.
• It is made up of only two factors: 1 and the digit itself.
• There is just one prime number that is even, which is 2.
• Some two prime numbers is always co-prime to one another.
• The product of prime numbers can be used to represent any integer.

Composite numbers

Composite numbers are whole numbers but with more than two elements, according to math.

Composite numbers are whole numbers that are divided by more than two digits but are not prime. The integers that can be formed by multiplying the two optimal value integers are also known as composite numbers. There has to be at least 1 divisor other than one in these integers. There are more than two composite elements in these figures. Any even number more than 2 is, for instance, a composite number.

Those few composite numbers, for examples, are 4, 6, 8, 9, and 10.

a composite example Because it can be divided by 1, 2, 3, 4, 6, and 12, the number 12 is a composite number. As a result, the number ’12’ includes six components.

• 19 /1 = 18; 18/2 = 9; 

• 18 / 3 Equals 6

• 18 / 9 equals 2

• 18 / 6 Equals 3

• 18/18 equals 1

Students should keep in mind that all values that aren’t prime can really be classified as composite numbers. These values can also be reduced by multiples of two. Six, for particular, is a composite number since it would be divided with 1, 2, 3, and 6.

Students are frequently asked to distinguish between prime and composite numbers. As a result, it is recommended that students fully comprehend the concepts of prime and composite numbers.

Another common concern students have about this concept would be whether zero belongs in the prime but rather composite number categories. The answers to these questions are that zero does not belong in either the prime or composites number categories.

Please remember what we’ve talked about thus far. Can you name all of the major composite numbers from one to two hundred? If you are unable to do so, please read the information following.

What Is the Best Way to Work Out Composite Numbers?

You must now comprehend the significance of consecutive composite numbers. You should also have a list of all the composite numbers ranging from 1 to 100 on hand. This is why it is now necessary to learn how to calculate the values of composite numbers. The procedure for calculating composite numbers is outlined in the next paragraph. Begin by identifying all of the positive integer’s factors. If a number contains only two elements, one and the number itself, it is referred to as a prime number. If there are more than two elements in a number, classify it as a composite number. Let’s say you’re trying to figure out whether 14 is a prime or composite number. To begin, you must first comprehend what prime and composite numbers are. After that, you must determine the 14 factors.

We already understand that 14 / 1 = 14.

7 = 14 / 2

2 = 14 / 7

1 = 14 / 14

Hence it is a composite number.

The Various Types of Composite Number

We’ll look at the many sorts of composite numbers in this section. Composite numbers are divided into two categories, according to specialists. The divisions are as follows:

Odd composite numbers, often known as composite odd numbers, are a type of odd composite number.

All composite numbers that are odd integers are called odd composite numbers. For instance, nine, fifteen, twenty-one, twenty-five, twenty-seven, and twenty-seven 

Even composites numbers, often known as composite even numbers, are a type of composite number.

Even composite numbers, on the other side, are all composite numbers that are also even numbers. In other words we can say, even composite numbers are even numbers that are not prime numbers. Even composite numbers, such as 4, 10, 16, 28, 56, and so on, exist. For instance, 4, 6, 8, and 10 are all numbers.

Composite Numbers vs. Prime Numbers

Also with help of the table below, we can better comprehend the difference among prime and composite numbers:

Prime Numbers	Composite Numbers
Values greater than 1 with only 1 and the number as its components.	At least three elements are present in numbers bigger than one.
The least but only even prime number is 2.	The smallest composite number is 4.
Prime numbers include 2, 3, 5, 7, 11, 13, and so on.	4, 6, 8, 9, 10, and so on are examples of composite numbers.
There is no even prime numbers except 2.	Composite numbers divided into two categories even and odd .

Chart of composite and prime number

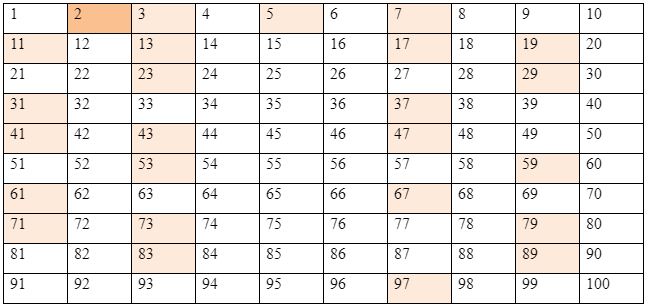
The highlighted boxes are prime numbers. We see 2 is the only even prime numbers.

Is There a Prime Number Pattern?

Following a pattern, you can find the set of prime numbers between any two numbers. The image below displays a few prime numbers surrounded, with all the digits divisible through these prime numbers struck out. This sequence can be repeated until you reach the square root of both the larger number, which is 100.

How do you determine whether a number is prime or composite?

There are a couple of tactics and ways for remembering whether a number is prime or composite. To determine whether a number is divisible by one or more numbers, we can use the division method and Divisibility Rules. To discover the prime factors of composite numbers, we can use the prime factorization method. Let’s see what we can do.

• A composite number is one that can be divided by another number (besides one).
• A composite number is any even number greater than two.
• A composite number is a number that is more than 2 and a multiple of 2.
• Similarly, any number other than 1 multiplied by it is not a prime number.
• It is a composite number if the prime factorization produces the product of the two or even more prime numbers.
• We will utilize divisibility rules to determine whether or not an integer is divisible by another number.

Find out what the smallest composite number.

The smallest composite value is four. This is because there are more than two components in the case of 4. The divisors of four are one, two, and four. You should also know that the smallest prime number is 2, the smallest even composite value is 9, and the smallest two component number is 12.

Numbers that are prime and those that are co-prime

There is a distinction to be made between prime and co-prime number. A pair of co-prime numbers is always considered, whereas a single number can be construed as a prime number. Co-prime numbers are made up of two numbers that have no common factor other than one. Co-prime numbers is either prime or composite, with the only requirement being that the GCF of co-prime values is always 1.

Co-prime numbers include the following:

The numbers 5 and 9 are co-primes.

The numbers 6 and 11 are co-primes.

Co-primes are 18 and 35.

It is not necessary for co-prime numbers to be prime numbers.

Here are some facts:

The number 2 is the only even prime number. Divide all other even numbers by two.

A number can be divided by three if the total of its digits is a multiple of three.

There isn’t a prime number bigger than 5 that don’t finish in a 5. Divide any integer larger than 5 that ends in a 5 by 5.

The numbers 0 and 1 are not prime numbers.

A number is either a prime number or a composite number, with the exception of 0 and 1. Any number greater than 1 that is not Prime is referred to as a composite number.

Importance of prime Numbers

Fewer people understand why these numbers are so essential, and how the mathematical logic that underpins them has led to critical applications in today’s society.

Here’s a fun fact about primes: Mathematicians have demonstrated that any whole number may be expressed entirely as a product of primes, and only primes.

Consider the following scenario:

Try 2 * 3 * 37 to get 222.

123,228,940? Simply put, 2 * 2 * 5 * 23 * 79 * 339

The Fundamental Theorem of Arithmetic is another name for this concept, which is known as the prime factorization rule. It makes sense when we consider prime numbers, which are numbers that cannot be separated any farther. So, if we try to break down each number into two numbers, then try to break them down into two numbers if feasible, and so on, we’ll end up with only primes.

This may appear to be nothing more than a fascinating mathematical anomaly. It becomes significant, however, because of one mere truth: it is completely hard to keep up with a highly effective formula for factoring huge numbers into primes, as far as the greatest mathematicians and computer scientists have indeed been able to determine.

As a result, the size of both the numbers we may factor into primes has a practical limit, and this fact is critical to modern digital security. Computer security is interested in pretty much anything that computers can accomplish without being able to undo quickly. Modern encryption techniques take advantage of the fact that we can easily multiply two enormous primes to generate a new, super-large number, but no machine has yet been constructed that can fast figure out where the two prime numbers went into producing it.

Such math-level security enables cryptographic algorithm, or encryption in which we don’t have to worry about publishing a key to encrypt communications because just holding that key (a very huge number) won’t help anybody undo the protection it established. You’ll need the prime factors of the key used for encryption to undo the encryption and read the message, which, as we’ve seen, isn’t something that you can find out with your own.

Hence,

Prime numbers are fun to play with. As Carl Sagan so eloquently points out in his novel Contact, their place as the most fundamental unit of all numbers, which are themselves the building blocks of our knowledge of the cosmos, has a particular significance. In that novel, aliens opt to deliver a long series of prime numbers as evidence that their communication is intelligent rather than natural, because prime numbers are the only thing that does not alter owing to variances in psychology, lifestyle, or human evolution. If an advanced alien life-form knows the universe around it, it very likely has the idea of a prime, regardless of how it appears or thinks.

Solved example 

Is 127 a composite or prime number?

Because 127 is an odd number, it cannot be divided by two.

According to the divisibility rule of three, if a number’s sum of digits is divisible by three, the number is also divisible by three.

1 + 2 + 7 = 10 = 127

The number ten is not divisible by three.

The number 127 is not divisible by five (since the unit place digit is not 0 or 5)

The number 127 is not divisible by seven (127/7 = 18.1425…)

(127/11 = 11.5454…) is not divisible by 11.

Similarly, we can divide 127 by other prime numbers such as 13, 17, 19, and so on.

Finally, we’ll discover that 127 is not divisible by any other number other than 1 and 127.

As a result, the number 127 is a prime number.