Area | Definition, Examples, Area Of Square, Triangle, Rectangle, Circle

Definition

The amount of space covered by a flat surface or piece of land or an object is called its area. The shaded part of each of the following figures shows the amount of space covered by each of them on a sheet of paper.

Units of measurement of Area

The units used to measure area are based on the units of length, i.e. mm, cm, m, km etc. to measure a region we use a square as a unit. Hence, the unit of area is a unit square. This is written as unit². Let us see who the different units are used to represent the area of a surface.

Unit Area

mm – mm² (1 mm² is equal to 1 square millimetres)

cm – cm²(1 cm² is equal to 1 square centimetres)

m – m² (1 m² is equal to 1 square metres)

km – km² (1 km² is equal to 1 square kilometres)

mi – mi² (1 mi² is equal to 1 square miles)

How to choose the correct unit when representing the area of a shape?

The unit for measuring the area depends on the size of the area being used. For example, the area, of the following are usually measured as under –

A Stamp – in m²

A notebook – in cm²

The floor of a room – in m²

A city – in km² or mi²

Let us understand the area with an example. Find out the areas of these shapes by counting the number of squares they cover. Give your answers in square units. Here we have some half squares as well. Count two half squares as 1 unit²

Number of full squares in this shape = 28

Number of half squares in this shape = 0

Area of the figure = 28 + 0 = 28 unit².

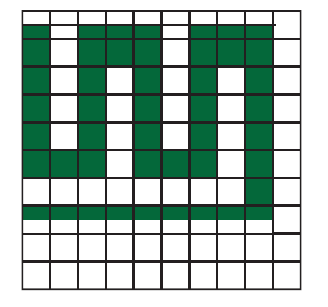
Number of full squares in this shape = 30

Number of half squares in this shape = 16

Area of the figure = 30 + $\frac{1}{2}$ x 16 = 30 + 8 = 38 unit².

Can shape having the same square units have different areas? Let us find out.

Consider the shapes below.

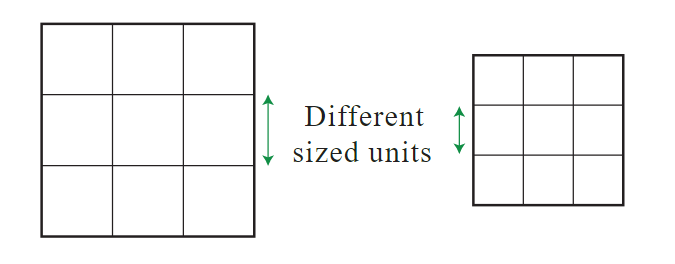
We can see that both shapes have 9 square units. However, the unit squares of both shapes differ in their sizes. This means that even though some shapes might have the same number of square units they may still differ in their areas.

Let us now discuss about the area of some common geometrical shapes.

Area of a Square

A square is a quadrilateral that has four equal sides and four right angles.

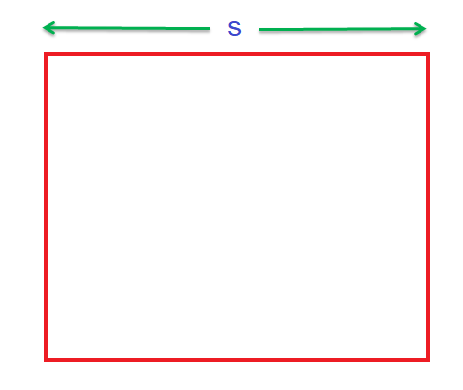
For a square whose side is of ‘s’ units –

Area of a Square = Side x Side = s² sq. units

For example, if we have a square whose one side is 6 cm, its area would be calculated as

Area = Side x Side = 6 x 6 = 36 cm²

Area of a Rectangle

A rectangle is a type of quadrilateral that equals opposite sides and four right angles.

Here we have a rectangle, covered with small squares. Each small square stands for 1 square centimetre, that is, each small square is cm on each side.

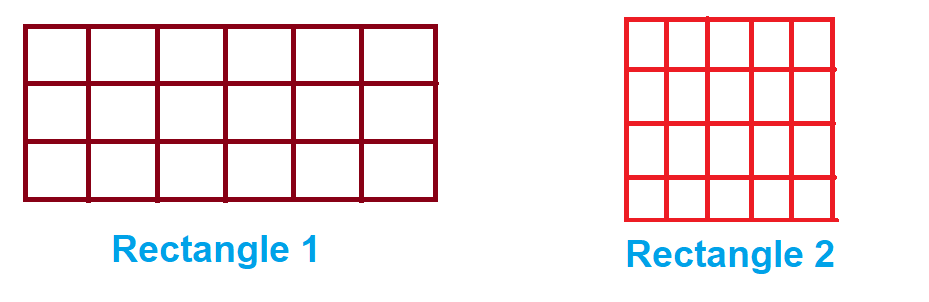
Count the number of squares that cover each rectangle. This will give you the area of a rectangle in square centimetres. Also, write the length and breadth of each rectangle. What do you observe? Let’s find out.

Rectangle 1

Number of small squares = 18

Area = 18 cm² or 18 sq. cm

Length = 6 cm

Breadth = 3 cm

Rectangle 2

Number of small squares = 20

Area = 20 cm² or 20 sq. cm

Length = 5 cm

Breadth = 4 cm

From the above examples, you can see that there is a quicker way to find the area in each case without having to count the number of squares. Since 6 x 3 = 18 and 5 x 4 = 50, we can say that the area of each of the two rectangles can be found out by multiplying the measures of length and breadth of the rectangle.

Area of a rectangle having length ‘l’ and breadth ‘b’ is given by l x b

For example, let us consider a rectangle having a length of 8 cm and a breadth of 7 cm as shown in the figure below.

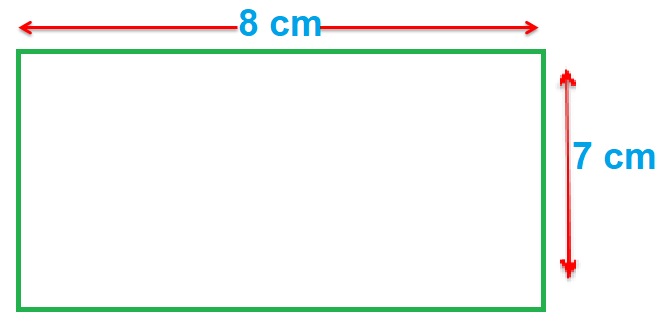
The area of this rectangle is given by 8 7 7 = 56 cm².

Hence

Area of a rectangle = length x breadth

Area of a Triangle

A triangle is a polygon that is made of three edges and three vertices. The vertices join together to make three sides of a triangle. The area occupied between these three sides is called the area of a triangle.

In general, the area of a triangle is defined by $\frac{1}{2}$ x b x h

Area of a triangle = $\frac{1}{2}$ x b x h

where b = base of the triangle (or any one side of the triangle), and

H = Height of the triangle from that base (or side)

The following figure illustrates the base and the height of a triangle

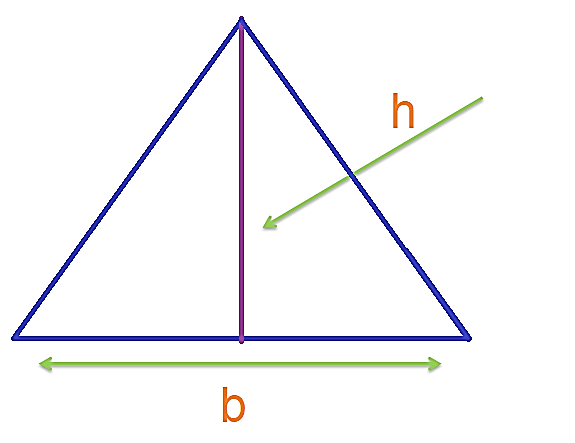
The above formula is applicable irrespective of the fact whether a triangle is a scalene triangle ( having different sides), an isosceles triangle ( having two sides equal), or an equilateral triangle ( having all sides equal).

Let us understand this more through an example. Suppose we have a triangle that has one side as 6 cm and an altitude (height) of 8 cm on that base as shown in the following figure –

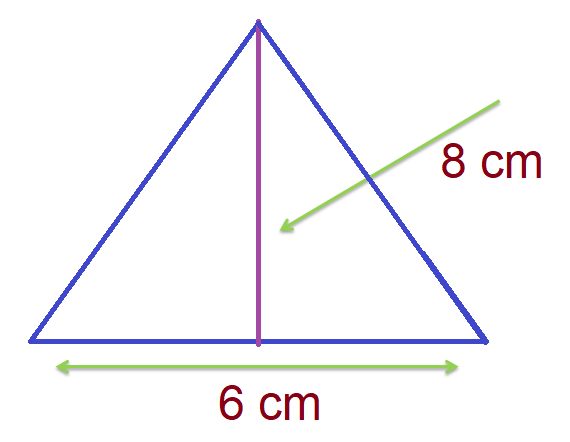
The area of this triangle is given by

$\frac{1}{2}$ x b x h

Where b = 6 cm and h = 8 cm

Therefore, Area = $\frac{1}{2}$ x 6 x 8 = 24 cm²

Area of a Circle

The space occupied by a circle is called its area.

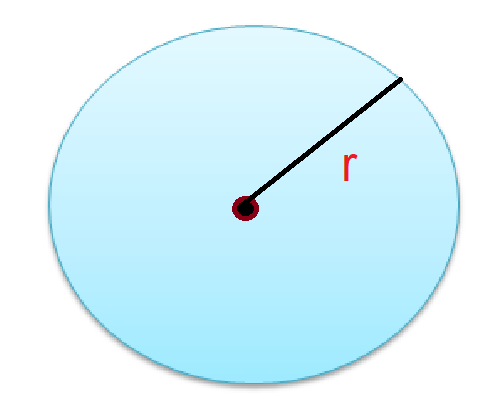
The area of a circle having a radius ‘r’ (The distance from the centre to a point on the boundary) is given by πr² where π = $\frac{22}{7}$ or 3.14 (approx.)

For example, suppose we have a circle that has a radius of 7 cm as shown in the figure below.

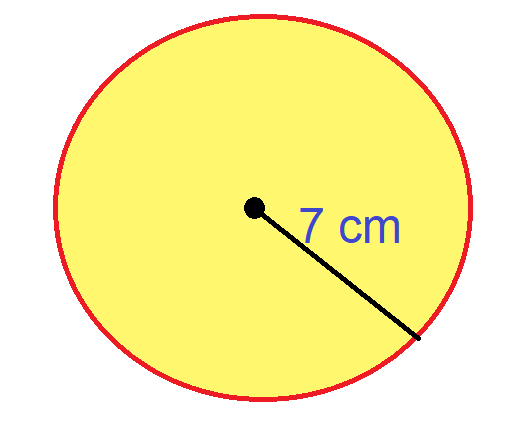
Its area is given by –

Area = πr² = $\frac{22}{7}$ x 7 x 7 = 154 cm²

Suppose, instead of the radius we are given the diameter of a circle, how do we calculate the area?

We know that in a circle, the radius is half of the diameter. Mathematically,

r = $\frac{d}{2}$, where ‘d’ is the diameter and ‘r’ is the radius.

So, we half the given diameter and obtain the radius.

Example

Suppose we are required to find the area of a circle having a diameter of 4.2 cm.

Here diameter (d) = 4.2 cm

By the relation between radius and diameter, we have, r = $\frac{d}{2}$

Hence r = $\frac{4.2}{2}$ = 2.1 cm

Now, area of this circle = = πr² = $\frac{22}{7}$ x 4.2 x 4.2 = 55.44 cm²

Area of a Parallelogram

A parallelogram is a quadrilateral, whose each pair of opposite sides is parallel. Suppose we have a parallelogram ABCD such that AB and DC are a pair of its opposite sides so that AB ‖ BC. Similarly, BC and AD are a pair of opposite sides such that BC ‖ AD.

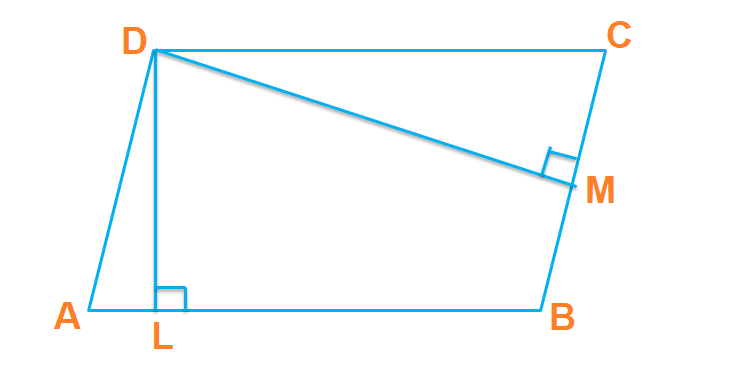
If DL ⟂AB, then any line, then we find any line segment with its end-points on the two sides AB and DC perpendicular to them has the length DL.so, we call AB as the base and DL the corresponding altitude.

Similarly, if DM ⟂BC, then any line segment with its end-points on the two sides AB and DC perpendicular to them has the length DM. so we can call BC as the base and DM as the corresponding altitude.

We have,

Area of a parallelogram = Base x Altitude

For example, let us find the area of a parallelogram having the base = 5 cm and altitude = 4.2 cm.

We know that, Area of a parallelogram = Base x Altitude

Here, base = 5 cm and altitude = 4.2 cm

Therefore,

Area = (5 x 4.2) cm^{2 =}21 cm²

Area of a Rhombus

Rhombus is a parallelogram with all its sides equal. Since the rhombus is a parallelogram with all its sides equal, therefore, the formula for the area of a parallelogram also holds true for calculating the area of a rhombus.

Hence

Area of a Rhombus = Base x Height

Example,

Let us have a rhombus whose altitude is 7 cm and the rhombus has a perimeter of 180 cm.

Solution

We are given that

The altitude of the rhombus = 7 cm

The perimeter of the rhombus = 180 cm.

We need to find the area of the rhombus. In order to do so, we must first find the side of the rhombus.

Now, remember that the perimeter of a closed shape is the sum of all its sides. Since the rhombus is a quadrilateral with all its sides equal, we can say that if the rhombus has a side “a”, then

a +a +a +a =180 cm

4a = 180 cm

a = 45 cm

Hence the side (base) of the rhombus = 45 cm

Now, to calculate the area of a rhombus, we know that

Area of a Rhombus = Base x Height

Therefore,

Area = (45 x 7) cm² = 315 cm²

Area of a Hexagon

A hexagon is a polygon having six sides. A regular hexagon is a hexagon that has its all sides equal.

The area of a regular hexagon can be calculated if we know one side of the hexagon. Let one side of a regular hexagon be “s”. Then the formula for calculating the area of a regular hexagon would be –

Area of a Hexagon = $\frac{3\sqrt{3}}{2}$s²

Example

Find the area of a regular hexagon, whose each side measures 6 cm.

Solution

To find the area of the hexagon, we need to know its side.

We are given that each side of the hexagon = 6 cm

Now, the area of a hexagon = $\frac{3\sqrt{3}}{2}$s²

So, area = $\frac{3\sqrt{3}}{2}$6² = $\frac{3\sqrt{3}}{2}$x 36 cm²= 54√3 cm²

Area of a Trapezium

A trapezium is a quadrilateral whose two sides are parallel.

Base – Each of the two sides of a trapezium is called the base of the trapezium.

Height or Altitude – The distance between the two bases (parallel lines) is called the height or altitude of the trapezium.

Below, we have the representation of the standard shape of a trapezium ABCD such that AB and CD are its parallel sides while AD and BC are its non-parallel sides.

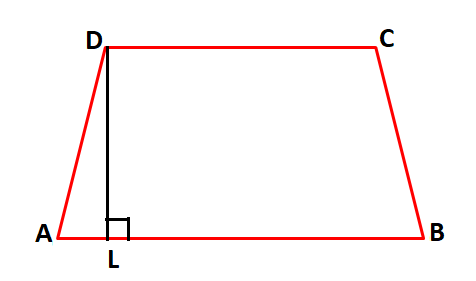
Let h be the height of the trapezium ABCDD. Then DL = h

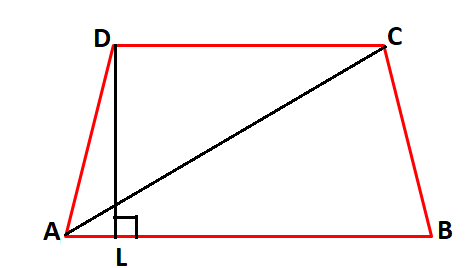
Area of trapezium ABCD = Area of ∆ABC + Area of ∆ACD

Area of trapezium ABCD = $\frac{1}{2}$ x AB x h + $\frac{1}{2}$ x DC x h

= $\frac{1}{2}$ x (AB + DC) x h

= $\frac{1}{2}$ x (Sum of parallel sides) x (Distance between parallel sides)

Hence,

Area of a trapezium = $\frac{1}{2}$ x (Sum of parallel sides) x (Distance between parallel sides)

Example

The area of a trapezium is 105 cm² and its height is 7 cm. If one of the parallel sides is longer than the other by 6 cm, find the two parallel sides.

Solution

We have been given that –

Area of the trapezium = 105 cm²

Height of the trapezium = = 7 cm

Also, one of the parallel sides is longer than the other by 6 cm.

Let one parallel side of the trapezium be p cm. Then, according to the question the other parallel side of the trapezium will be (p + 6) cm

Now, we know that

Area of a trapezium = $\frac{1}{2}$ x (Sum of parallel sides) x (Distance between parallel sides)

Therefore, putting the values we have in the above equation we get,

105 = 12 x (p + p + 6) x 7

⇒105 x 2 = (2p + 6) x 7

⇒ 210 = 14p + 42

⇒14p = 210 – 42 = 168

⇒ p = $\frac{168}{14}$ = 12 cm

Therefore, one parallel side of the trapezium = 12 cm.

The other parallel side of the trapezium = (12 + 6) cm = 18 cm

Hence, the two parallel sides of the trapezium are 12 cm and 18 cm

We know that a net is a two-dimensional shape that can be folded to make a three-dimensional shape. Can we use nets to find the area of a geometric shape? Let us find out.

How can nets be used to determine area?

We know now that a net is a pattern made when the surface of a three-dimensional figure is laid out flat showing each face of the figure. How can we use the nets to find the area of a 3 – dimensional object? Net allows us to see all the 2-dimensional objects that make a 3 – dimensional object. The following steps are used to find the area of a 3 – dimensional object using nets –

The first step is to decompose the 3 – dimensional object into rectangles, triangles, circles, squares and label each of the 2-dimensional shapes thus obtained.
The next step is to find the dimensions which are the lengths of the sides that would be required to find the area.
Now that we have the dimensions of each side, we can find out the area of each 2-dimensional shape that we had obtained in the first step.
After fining the area of all the 2-dimensional shapes, the last step is to add up all the area to get the total area which is the area of the given 3 – dimensional shape.

Let us understand it using an example

How can we find the area of a cube using nets? There can be 11 different possible nets of a cube. Below are the various nets possible –

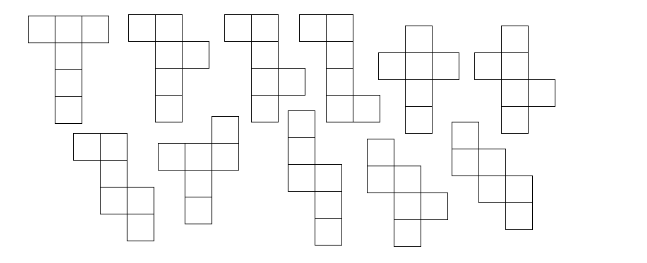
This is important to understand so as to have clarity on the 3 – dimensional shapes that has been unfolded so as to form a structure of nets. In the same manner, we can use nets to find areas of other geometric shapes such as prisms.

Key Facts and Summary

The amount of space covered by a flat surface or piece of land or an object is called its area.
Area of a Square = Side x Side = s² sq. units
Area of a rectangle = length x breadth
The area of a circle having a radius ‘r’ (The distance from the centre to a point on the boundary) is given by πr² where π = $\frac{22}{7}$ or 3.14 (approx.)
Area of a triangle = $\frac{1}{2}$ x b x h, where b = base of the triangle (or any one side of the triangle), and h = Height of the triangle from that base (or side)
Area of a parallelogram = Base x Altitude
Area of a Rhombus = Base x Height
Area of a Hexagon = $\frac{3\sqrt{3}}{2}$s²
Area of a trapezium = $\frac{1}{2}$ x (Sum of parallel sides) x (Distance between parallel sides)
A net is a pattern made when the surface of a three-dimensional figure is laid out flat showing each face of the figure. Nets can be used to find the area of 3 dimensional shapes.

Recommended Worksheets

Area of a Trapezoid (Arbor Day Themed) Math Worksheets
Area of a Rhombus (April Fool’s Day Themed) Math Worksheets
Area of a Parallelogram (Earth Day Themed) Math Worksheets

Browse All Worksheets

We spend a lot of time researching and compiling the information on this site. If you find this useful in your research, please use the tool below to properly link to or reference Helping with Math as the source. We appreciate your support!