Home » Math Theory » Algebra » Addition & Subtraction Of Rational Expression

Addition & Subtraction Of Rational Expression

Introduction

We are familiar with the term algebraic expressions. Recall that an algebraic expression (or) a variable expression is a combination of terms by the operations such as addition, subtraction, multiplication, division, etc. an extension to the algebraic expressions are the polynomials. Also, an algebraic expression, in which variable(s) does (do) not occur in the denominator, exponents of variable(s) are whole numbers and numerical coefficients of various terms are real numbers, is called a polynomial. So what do we mean by a rational expression? Is it the same as an algebraic expression or a polynomial, or it has a different meaning to it? Let us find out.

What is a Rational Expression?

Rational expressions are fractions that have a polynomial in the numerator, denominator, or both. For example, $\frac{x}{x-2}$ is a rational expression as it has polynomials in both its numerator as well as its denominator. Before we start understanding how to add or subtract rational expressions, it is important to recall some important terms and concepts that are integral to rational expressions. 

Factors are the building blocks of multiplication. In the case of rational expressions as well, factors are those algebraic expressions that completely divide the rational expression. For example, the factor of the rational expression $\frac{x^2-1}{x+1}$ will be x + 1 as it is common to both the numerator as well as the denominator. 

Therefore, a number or an algebraic expression can be said to be a factor of a rational expression, if it is common to both the numerator as well as its denominator. 

Terms are single numbers or variables and numbers connected by multiplication. For example, 6, -5, x3 are all terms.

Expressions are groups of terms connected by addition and subtraction. For example, 4x2 – 7 is an expression.

Simplifying Rational Expressions

Simplification of a rational expression is the process of reducing a rational expression in its lowest terms possible. It is similar to reducing fractions or rational numbers to their lowest form. The following steps are sued for simplification of rational expressions-

  1. Factorize both the denominator and numerator of the rational expression. Also, remember to write each expression in standard form. 
  2. Reduce the expression by cancelling out common factors in the numerator and denominator
  3. Rewrite the remaining factors in the numerator and denominator.

Let us understand the above steps by an example.

Example

Simplify the rational expression $\frac{3x^2- 3x}{3x^3- 6x^2+3x}$

Solution

We are given the rational expression

$\frac{3x^2- 3x}{3x^3- 6x^2+3x}$

In order to simplify this rational expression, first we will look for factors that are common to the numerator & denominator. We have, 3x is a common factor the numerator & denominator. Note that it is clear that x ≠0. Hence we will cancel the common factor to get,

$\frac{3x(x-1)}{3x(x^2-2x+1)}$

Now, if possible, we will look for other factors that are common to the numerator and denominator. We can see that ( x – 1 ) is also a common factor of the numerator and the denominator as x2-2x+1 can be written as ( x – 1 ) ( x – 1 ) .

Therefore, we now have,

$\frac{(x-1)}{(x-1)(x-1)}$

We now have,

$\frac{1}{( x-1 )}$

Therefore, the rational expression $\frac{3x^2- 3x}{3x^3- 6x^2+3x}$ in its simplified form will be $\frac{1}{( x-1 )}$

Let us now learn how to add and subtract rational expressions. 

How to add and subtract Rational Expressions? 

The addition and subtraction of rational expression is done in the same manner as we do for fractions. This means that for adding and subtracting rational expressions depends whether they have same or different denominators.  But an important point to be noted here is that you can add or subtract rational expressions only when you have a common denominator. Let us consider both the cases one by one.

Rational Expressions having the Same Denominators

The following steps should be followed while adding or subtracting rational expressions having the same denominators – 

  1. Obtain the numerators of the two given rational expressions and their common denominator
  2. Add the numerators obtained in the first step.
  3. Write a rational expression whose numerator is the sum obtained in the second step and the denominator is the common denominator of the given rational expressions.

Let us understand the above steps with an example.

Example

Solve the rational expressions $\frac{x}{x-1} + \frac{2-x}{x-1}$

Solution

We are required to find the value of $\frac{x}{x-1} + \frac{2-x}{x-1}$

We can clearly see that both the rational expressions have the same denominator, i.e. x – 1.

Therefore, going by the above stated steps, we just need to add the numerators of the two rational expressions given to us. We will then have,

$\frac{x}{x-1} + \frac{2-x}{x-1} = \frac{x+2-x}{x-1} = \frac{2}{x-1}$

Hence, $\frac{x}{x-1} + \frac{2-x}{x-1} = \frac{2}{x-1}$

Now, let us see how we will add or subtract rational expressions have different denominators.

Rational Expressions having Different Denominators

For rational expressions having different denominators, we cannot add them in the same manner as we did for rational expressions having same denominator, where we simply added or subtracted the numerators. In order to add or subtract two or more rational expressions with different denominators, the following steps will be followed – 

  1. Obtain the rational expressions and their denominators. The denominators of the fractions should be such that they are not the same. 
  2. Find the Least Common Multiple ( L.C.M) of the denominators. In other words, make the denominators the same by finding the Least Common Multiple (LCM) of their denominators. This step is exactly the same as finding the Least Common Denominator (LCD).
  3. Multiply each fraction by the appropriate form of the L.C.M so as to obtain equivalent rational expressions with same denominators. 
  4.  Reduce the resultant rational expression if possible.
  5. Let us understand the above steps with an example.

Example

Solve    $\frac{x+7}{2x+12} + \frac{6}{x^2- 36}$

Solution

We are required to find the value of $\frac{x+7}{2x+12} + \frac{6}{x^2- 36}$

To solve this, we will follow the above stated steps. First of all we will find a common denominator of the given rational expressions. To find a common denominator, start by factoring the denominators of both fractions. Therefore,

$\frac{x+7}{2x+12} + \frac{6}{x^2- 36} = \frac{x+7}{2(x+6)} + \frac{6}{(x+6)(x-6)}$

From above, we can see that the least common denominator of these two fractions is 

2 ( x + 6 ) ( x – 6 )

Looking at the first rational expression, we can see that the factor missing from its denominator is the rational expression ( x – 6 ). Therefore, in the next step, we’ll multiply the top and bottom of the first rational expression by (x-6). Similarly, if we look at the second rational expression, we can see that the factor missing from its denominator is 2. In the next step, we’ll multiply the top and bottom of the second rational expression by 2. We will now get,

$\frac{x+7}{2(x+6)} + \frac{6}{(x+6)(x-6)} = \frac{(x+7)(x-6)}{2(x+6)} + \frac{6 x 2}{(x+6)(x-6)}$

Now that the fractions have the same denominators, we’ll create one combined fraction by adding the two fractions together, to get,

$\frac{(x+7)(x-6)}{2(x+6)} + \frac{6 x 2}{(x+6)(x-6)} = \frac{(x+7)(x-6)+12}{2(x+6)( x-6 )}$

Now, we will simplify the top of the rational expression we have now obtained. Notice that we are only simplifying the top of the rational expression; the bottom of the rational expression remains unchanged.

$\frac{(x+7)(x-6)+12}{2(x+6)( x-6 )} = \frac{x^2+7x-6x-42+12}{2(x+6)(x-6)}$

=$\frac{x^2+x-30}{2(x+6)(x-6)} = \frac{(x-5)( x+6)}{2(x+6)(x-6)}$

Now that we have obtained the rational expression, we will reduce it to obtain its simplified form.

So, on reducing the rational expression to its lowest from, we get

 $\frac{(x-5)( x+6)}{2(x+6)(x-6)} = \frac{(x-5)(x+6)}{2(x+6)(x-6)} = \frac{(x-5)}{2(x+6)}$

Hence, $\frac{x+7}{2x+12} + \frac{6}{x^2- 36} = \frac{(x-5)}{2(x+6)}$

Let us summarise the steps that have been discussed above for addition ro subtraction of rational expressions.

Adding or subtracting rational expressions is a four-step process:

  1. Write all fractions as equivalent fractions with a common denominator.
  2. Combine the fractions as a single fraction that has the common denominator.
  3. Simplify the expression in the top of the fraction.
  4. Reduce the fraction to lowest terms.

General Rules for Addition or Subtraction of Rational Expressions

While adding or subtracting rational expression, the following rules must be kept in mind – 

  1. Rational expressions (fractions) can only be added or subtracted if they have a common denominator.
  2. The numerator and denominator of a fraction may be multiplied by the same quantity. This will result in a fraction that is equivalent to the original fraction.
  3. For a fractional answer to be in final form, the fraction must be reduced to lowest terms.
  4. When subtracting rational expressions, note that the entire numerator of the second fraction and not just the first term must be subtracted. Also note that the sign of each term of the numerator being subtracted will change when the parentheses are removed.

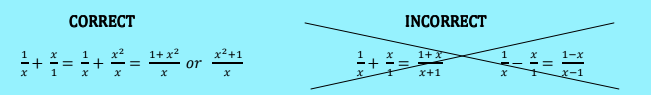
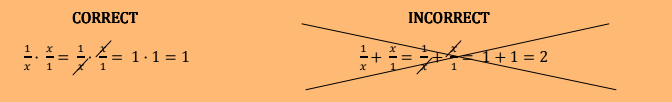
Avoiding Common Errors

Here is a list of some errors that we usually commit during addition or subtraction of rational expressions – 

  1. A common error in an addition or subtraction problem is to add or subtract the numerators and the denominators. So, you cannot just the numerator with the denominator and the denominator with the denominator.
  2. Remember that to add or subtract fraction, you must first have a common denominator. Then you add or subtract the numerators while maintaining the common denominator. Another common mistake is to treat an addition or subtraction problem as a multiplication problem. You can divide out common factors only when multiplying expressions, not when adding or subtracting them.

Solved Examples

Example 1 Solve $\frac{4x+1}{x^2+5x+4} – \frac{x+3}{x^2+4x+3}$

Solution We are required to find the value of $\frac{4x+1}{x^2+5x+4} – \frac{x+3}{x^2+4x+3}$

To solve this, we will follow the steps we have learnt above for addition and subtraction of rational expressions. First of all we will find a common denominator of the given rational expressions. To find a common denominator, start by factoring the denominators of both rational expressions. Therefore we have,

$\frac{4x+1}{x2+5x+4} – \frac{x+3}{x^2+4x+3} = \frac{4x+1}{(x+4)(x+1)} – \frac{(x+3)}{(x+1)(x+3)}$

After factoring both the rational expression, we can see that the least common denominator of these two rational expressions is (x+4)(x+1)(x+3). Looking at the rational expression, we can see that the factor missing from its denominator is (x+3). In the next step, we’ll multiply the top and bottom of the first rational expression by (x+3). Similarly, looking at the second rational expression, we can see that the factor missing from its denominator is (x+4). In the next step, we’ll multiply the top and bottom of the second rational expression by (x+4).

Therefore, we will now have,

$\frac{4x+1}{(x+4)(x+1)} – \frac{x+3}{(x+1)(x+3)} = \frac{(4x+1)(x+3)}{(x+4)(x+1)(x+3)} – \frac{(x+3)(x+4)}{(x+4)(x+1)(x+3)}$

Now that the rational expressions have the same denominators, we’ll create one combined rational expression by subtracting the rational expressions. We will now get,

$\frac{(4x+1)(x+3)}{(x+4)(x+1)(x+3)} – \frac{(x+3)(x+4)}{(x+4)(x+1)(x+3)} = \frac{(4x+1)(x+3)-(x+3)(x+4)}{(x+4)(x+1)(x+3)}$

 We will now simplify the top of the rational expression. Notice that we are only simplifying the top of the rational expression; the bottom of the rational expression remains unchanged. We will get,

$\frac{(4x+1)(x+3)-(x+3)(x+4)}{(x+4)(x+1)(x+3)} = \frac{4x^2+x+12x+3-(x^2+3x+4x+12)}{(x+4)(x+1)(x+3)}$

= $\frac{4x^2+x+12x+3-x^2+3x+4x+12}{(x+4)(x+1)(x+3)}$

= $\frac{3x^2+6x-9}{(x+4)(x+1)(x+3)}$

= $\frac{3(x^2+2x-3)}{(x+4)(x+1)(x+3)}$

= $\frac{3(x+3)(x-1)}{(x+4)(x+1)(x+3)}$

Now that we have obtained the rational expression, we will reduce it to obtain its simplified form.

So, on reducing the rational expression to its lowest form, we get

$\frac{3(x+3)(x-1)}{(x+4)(x+1)(x+3)} = \frac{3(x+3)(x-1)}{(x+4)(x+1)(x+3)} = \frac{3(x-1)}{(x+4)(x+1)}$

Hence, $\frac{4x+1}{x^2+5x+4} – \frac{x+3}{x^2+4x+3} = \frac{3(x-1)}{(x+4)(x+1)}$

Example 2 Find the value of $\frac{1}{x^2-4} – \frac{1}{2-x}$

Solution We are required to find the value of $\frac{1}{x^2-4} – \frac{1}{2-x}$

To solve this, we will follow the steps we have learnt above for addition and subtraction of rational expressions. First of all we will find a common denominator of the given rational expressions. To find a common denominator, start by factoring the denominators of both rational expressions. Therefore we have,

$\frac{1}{x^2-4} – \frac{1}{2-x} = \frac{1}{(x+2)(x-2)} – \frac{1}{-1(x-2)} = \frac{1}{(x+2)(x-2)} + \frac{1}{(x-2)}$

After factoring both the rational expression, we can see that the least common denominator of these two rational expressions is  ( x + 2 ) ( x – 2 ). Looking at the first rational expression, we can see that its denominator is the exact value of the L.C.M, so we need not make any changes to it. Similarly, looking at the second rational expression, we can see that the factor missing from its denominator is ( x + 2 ). In the next step, we’ll multiply the top and bottom of the second rational expression by ( x  + 2 ). We will have,

$\frac{1}{(x+2)(x-2)} + \frac{1}{(x-2)} = \frac{1}{(x+2)(x-2)} + \frac{(x+2)}{(x-2)(x+2)}$

Now that the rational expressions have the same denominators, we’ll create one combined rational expression by subtracting the rational expressions. We will now get,

$\frac{1}{(x+2)(x-2)} + \frac{(x+2)}{(x-2)(x+2)} = \frac{1+(x+2)}{(x+2)(x-2)} = \frac{x+3}{(x+2)(x-2)}$

Now that we have obtained the rational expression, we will reduce it to obtain its simplified form. But, we can see that in the obtained rational expression, there are no common factors between the numerator and the denominator. Hence it is already in its simplest form.  

Therefore, 

$\frac{1}{x^2-4} – \frac{1}{2-x} = \frac{x+3}{(x+2)(x-2)}$

Key Facts and Summary

  1. An algebraic expression (or) a variable expression is a combination of terms by the operations such as addition, subtraction, multiplication, division, etc.
  2. An algebraic expression, in which variable(s) does (do) not occur in the denominator, exponents of variable(s) are whole numbers and numerical coefficients of various terms are real numbers, is called a polynomial.
  3. Rational expressions are fractions that have a polynomial in the numerator, denominator, or both.
  4. A number or an algebraic expression can be said to be a factor of a rational expression, if it is common to both the numerator as well as its denominator.
  5. Terms are single numbers, or variables and numbers connected by multiplication. 6, -5, x3 are all terms.
  6. Expressions are groups of terms connected by addition and subtraction. 4x2 – 7 is an expression.
  7. Adding or subtracting rational expressions is a four-step process. Write all fractions as equivalent fractions with a common denominator. Combine the fractions as a single fraction that has the common denominator. Simplify the expression in the top of the fraction. Reduce the fraction to the lowest terms.
  8. Rational expressions (fractions) can only be added or subtracted if they have a common denominator.
  9. The numerator and denominator of a fraction may be multiplied by the same quantity. This will result in a fraction that is equivalent to the original fraction.
  10. For a fractional answer to be in final form, the fraction must be reduced to the lowest terms.
  11. When subtracting rational expressions, note that the entire numerator of the second fraction and not just the first term must be subtracted. Also note that the sign of each term of the numerator being subtracted will change when the parentheses are removed.

Properties of Addition (National Sandwich Day Themed) Math Worksheets
Addition of Decimals (Bank/Money Themed) Worksheets
Addition of RAEs with Different Denominators (Vacation Themed) Math Worksheets

Browse All Worksheets

Link/Reference Us

We spend a lot of time researching and compiling the information on this site. If you find this useful in your research, please use the tool below to properly link to or reference Helping with Math as the source. We appreciate your support!

  • "Addition & Subtraction Of Rational Expression". Helping with Math. Accessed on April 25, 2024. https://helpingwithmath.com/addition-subtraction-of-rational-expression/.

  • "Addition & Subtraction Of Rational Expression". Helping with Math, https://helpingwithmath.com/addition-subtraction-of-rational-expression/. Accessed 25 April, 2024.

  • Addition & Subtraction Of Rational Expression. Helping with Math. Retrieved from https://helpingwithmath.com/addition-subtraction-of-rational-expression/.