What is a mixed number?
A mixed number is a number expressed as the combination of a whole number and fraction. In general, it denotes a value between two whole numbers.
Consider the mixed number $2\frac{3}{5}$.
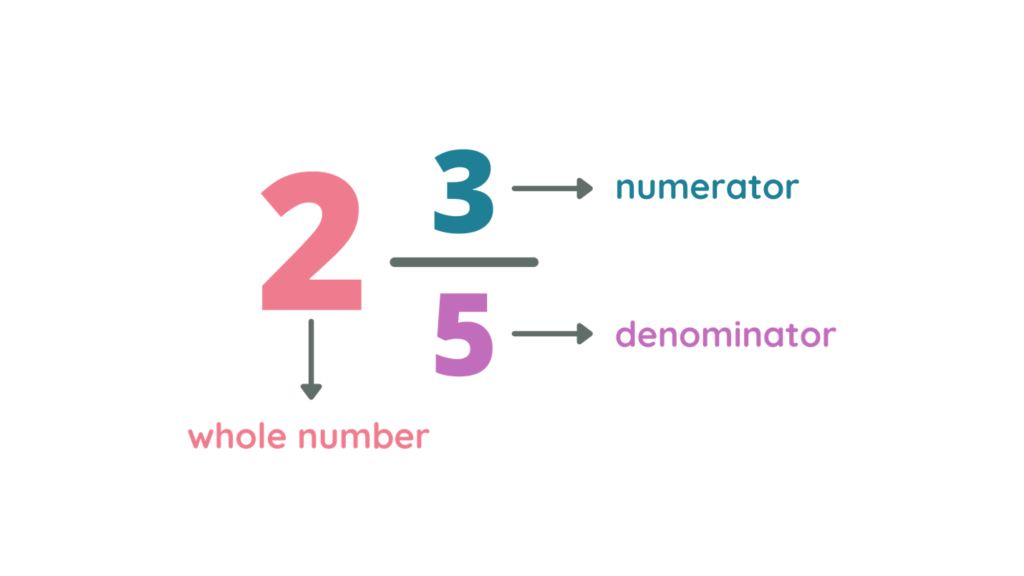
In this example,
- 2 is the whole number
- 3 is the numerator of the fraction; and
- 5 is the denominator of the fraction.
How to convert an improper fraction to a mixed number?
An improper fraction is a fraction that has a numerator greater than the denominator. Improper fractions can be converted into mixed numbers.
Consider the fraction $\frac{21}{8}$.
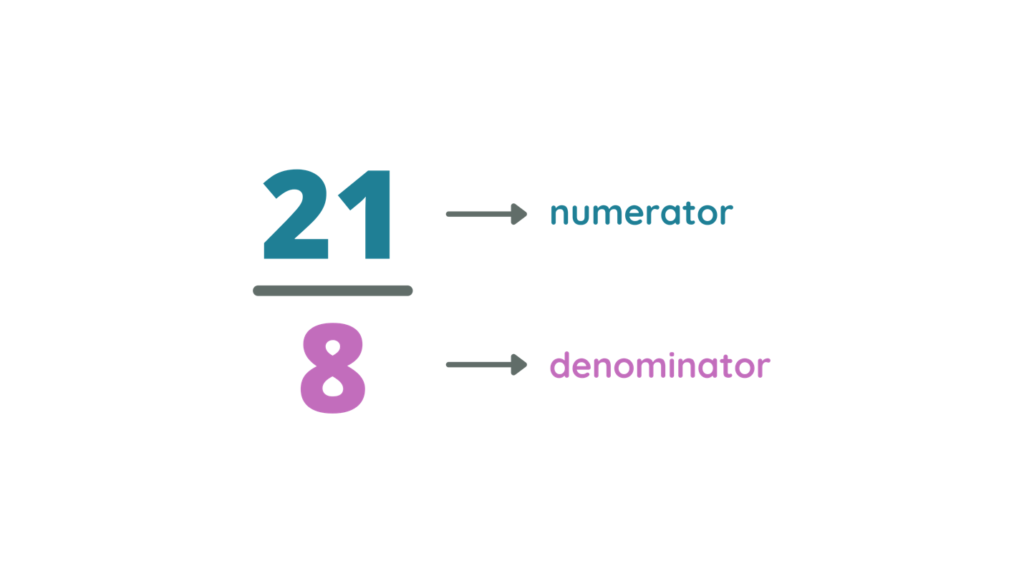
Since the numerator is greater than the denominator, it is considered as an improper fraction.
To convert improper fractions to mixed numbers:
- Divide the numerator by the denominator.
- Write the whole number.
- Write the remainder as the numerator.
- Write the same denominator.
Example #1
Convert $\frac{11}{2}$ to a mixed number.
Solution
| Process | Step-by-step Explanation |
| 11÷2 = 5 with a remainder of 1 | Divide the numerator by the denominator. In this case, the numerator is 11 and the denominator is 2. |
| $5\frac{1}{}$ | Write the whole number as the whole number of the mixed number and write the remainder as the numerator of the fraction. |
| $5\frac{1}{2}$ | Copy the denominator of the given improper fraction. |
| Therefore, $\frac{11}{2}$ will become $5\frac{1}{2}$ if converted as a mixed number. |
Example #2
Convert $\frac{27}{7}$ to a mixed number.
Solution
| Process | Step-by-step Explanation |
| 27÷7 = 3 with a remainder of 6 | Divide the numerator by the denominator. In this case, the numerator is 27 and the denominator is 7. |
| $3\frac{6}{}$ | Write the whole number as the whole number of the mixed number and write the remainder as the numerator of the fraction. |
| $3\frac{6}{7}$ | Copy the denominator of the given improper fraction. |
| Therefore, $\frac{27}{7}$ will become $3\frac{6}{7}$ if converted as a mixed number. |
How to convert a mixed number to an improper fraction?
Converting mixed numbers to an improper fraction is one step that you need to know in adding and subtracting mixed numbers.
To convert mixed numbers to improper fractions:
- Multiply the denominator with the whole number.
- Add the numerator to the product of the denominator and whole number.
- Write the sum on the numerator of the improper fraction.
- Copy the same denominator.
Example #1
Convert $6\frac{6}{13}$ to an improper fraction.
Solution
| Process | Step-by-step Explanation |
| 6 x 13 = 78 | Multiply the denominator to the given whole number of the mixed number. |
| 78 + 6 = 84 | Add the product to the numerator of the mixed number. |
| $\frac{84}{}$ | Write the sum as the numerator of the improper fraction. |
| $\frac{84}{13}$ | Copy the denominator of the given mixed number. |
| Therefore, $6\frac{6}{13}$ will become $\frac{84}{13}$ if converted as a mixed number. |
Example #2
Convert $2\frac{7}{11}$ to an improper fraction.
Solution
| Process | Step-by-step Explanation |
| 2 x 11 = 22 | Multiply the denominator to the given whole number of the mixed number. |
| 22 + 7 = 29 | Add the product to the numerator of the mixed number. |
| $\frac{29}{}$ | Write the sum as the numerator of the improper fraction. |
| $\frac{29}{11}$ | Copy the denominator of the given mixed number. |
| Therefore, $2\frac{7}{11}$ will become $\frac{29}{11}$ if converted as a mixed number. |
How to add mixed numbers?
When adding mixed numbers, we need to consider the addends and the denominators of the addends.
Adding whole number and mixed number
To add a whole number and a mixed number, follow these steps:
- Add the given whole number and the whole number of the mixed number.
- Copy the fraction part of the mixed number on the final answer.
Example #1
What is the sum of 2 and $3\frac{1}{4}$?
Solution
| Addition Process | Step-by-step Explanation |
| $2+3\frac{1}{4} =$ | Set up the addition process. |
| $2+3\frac{1}{4} =5$ | Add the whole numbers 2 and 3. |
| $2+3\frac{1}{4} =5\frac{1}{4}$ | Copy the fraction part of the given in mixed numbers. |
| Therefore, the sum of 2 and $3\frac{1}{4}\:is\:5\frac{1}{4}$. |
Example #2
Determine the sum of $10\frac{4}{7}$ and 6.
Solution
| Addition Process | Step-by-step Explanation |
| $10\frac{4}{7} + 6$ | Set up the addition process. |
| $10\frac{4}{7} + 6 = 16$ | Add the whole numbers 10 and 36. |
| $10\frac{4}{7} + 6 = 16\frac{4}{7}$ | Copy the fraction part of the given in mixed numbers. |
| Therefore, the sum of $10\frac{4}{7}$ and 6 is $16\frac{4}{7}$. |
Adding mixed numbers with the same denominator
To add mixed numbers with the same denominators, follow these steps:
- Convert the mixed number to an improper fraction.
- Add the numerators.
- Copy the denominator.
- Convert the improper fraction to a mixed number.
Example #1
What is the sum of $3\frac{2}{5}$ and $7\frac{4}{5}$?
Solution
| Addition Process | Step-by-step Explanation |
| $3\frac{2}{5} + 7\frac{4}{5} =$ | Set up the addition process. |
| $3\frac{2}{5}$ ⟹ $\frac{17}{5}$ | Convert $3\frac{2}{5}$ to an improper fraction. |
| $7\frac{4}{5}$ ⟹ $\frac{39}{5}$ | Convert $7\frac{4}{5}$ to an improper fraction. |
| $\frac{17}{5} + \frac{39}{5} =$ | Set up the addition process. |
| $\frac{17}{5} + \frac{39}{5} =\frac{56}{}$ | Add the numerators. |
| $\frac{17}{5} + \frac{39}{5} =\frac{56}{5}$ | Copy the denominator. |
| $\frac{56}{5}$ ⟹$11\frac{1}{5}$ | Convert $\frac{56}{5}$ to a mixed number. |
| Therefore, the sum of $ 3\frac{2}{5}$ and $7\frac{4}{5}$ is $11\frac{1}{5}$. |
Example #2
Determine the sum of $6\frac{5}{7}$ and $3\frac{3}{7}$.
Solution
| Addition Process | Step-by-step Explanation |
| $6\frac{5}{7} + 3\frac{3}{7} = $ | Set up the addition process. |
| $6\frac{5}{7}$ ⟹ $\frac{47}{7}$ | Convert $6\frac{5}{7}$ to an improper fraction. |
| $3\frac{3}{7}$ ⟹ $\frac{24}{7}$ | Convert $3\frac{3}{7}$ to an improper fraction. |
| $\frac{47}{7} + \frac{24}{7} =$ | Set up the addition process. |
| $\frac{47}{7} + \frac{24}{7} = \frac{71}{}$ | Add the numerators. |
| $\frac{47}{7} + \frac{24}{7} = \frac{71}{7}$ | Copy the denominator. |
| $\frac{71}{7}$⟹ $10\frac{1}{7}$ | Convert $\frac{71}{7}$ to a mixed number. |
| Therefore, the result of adding $6\frac{5}{7}$ and $3\frac{3}{7}$ is $10\frac{1}{7}$. |
Example #3
Find the result of $13\frac{2}{3} + 6\frac{1}{3}$.
Solution
| Addition Process | Step-by-step Explanation |
| $13\frac{2}{3} + 6\frac{1}{3} =$ | Set up the addition process. |
| $13\frac{2}{3}$ ⟹ $\frac{41}{3}$ | Convert $13\frac{2}{3}$ to an improper fraction. |
| $6\frac{1}{3}$ ⟹ $\frac{19}{3}$ | Convert $6\frac{1}{3}$ to an improper fraction. |
| $\frac{41}{3} + \frac{19}{3} =$ | Set up the addition process. |
| $\frac{41}{3} + \frac{19}{3} = \frac{60}{}$ | Add the numerators. |
| $\frac{41}{3} + \frac{19}{3} = \frac{60}{3}$ | Copy the denominator. |
| $\frac{60}{3}$ ⟹ 20 | Convert 60 3 to a mixed number. |
| Therefore, the sum of $13\frac{2}{3}$ and $6\frac{1}{3}$ is 20. |
Example #4
What is the sum of $5\frac{1}{2}$ and $\frac{7}{2}$?
Solution
| Addition Process | Step-by-step Explanation |
| $5\frac{1}{2} + \frac{7}{2} =$ | Set up the addition process. |
| $5\frac{1}{2}$ ⟹ $\frac{11}{2}$ | Convert $5\frac{1}{2}$ to an improper fraction. |
| $\frac{11}{2} + \frac{7}{2} = $ | Set up the addition process. |
| $\frac{11}{2} + \frac{7}{2} = \frac{18}{}$ | Add the numerators. |
| $\frac{11}{2} + \frac{7}{2} = \frac{18}{2}$ | Copy the denominator. |
| $\frac{18}{2}$ ⟹ 9 | Convert $\frac{18}{2}$ to a mixed number. |
| Therefore, the sum of $5\frac{1}{2}\:and\:\frac{7}{2}$ is 9. |
Adding mixed numbers with different denominators
To add mixed numbers with different denominators, follow these steps:
- Convert the mixed number to an improper fraction.
- Find the least common denominator (LCD).
- Divide the LCD by the original denominators of the given mixed numbers.
- Multiply the quotient to the denominator of the improper fraction.
- Write the fractions based on the LCD.
- Add the numerators.
- Copy the LCD.
- Convert the improper fraction to a mixed number.
Example #1
What is the sum of $4\frac{1}{2}$ and $\frac{3}{4}$?
Solution
| Addition Process | Step-by-step Explanation |
| $4\frac{1}{2} + \frac{3}{4} =$ | Set up the addition process. |
| $4\frac{1}{2}$ ⟹ $\frac{9}{2}$ | Convert $4\frac{1}{2}$ to an improper fraction. |
| $\frac{9}{2} + \frac{3}{4} =$ | Set up the addition process. |
| $\frac{9}{2}$ ⟹ $\frac{18}{4}$ $\frac{3}{4}$ ⟹ $\frac{3}{4}$ | Find the least common denominator of 2 and 4. By prime factorization, the least common multiple of 2 and 4 is 4. Hence, we will divide the LCD to the original denominator and multiply the quotient to the numerator. |
| $\frac{18}{4} + \frac{3}{4} =$ | Set up the addition process. |
| $\frac{18}{4} + \frac{3}{4} =\frac{21}{}$ | Add the numerators. |
| $\frac{18}{4} + \frac{3}{4} =\frac{21}{4}$ | Copy th4e denominator. |
| $\frac{21}{4}$⟹ $5\frac{1}{4}$ | Convert \frac{21}{4}$ to a mixed number. |
| Therefore, the sum of $4\frac{1}{2}$ and $\frac{3}{4}$ is $5\frac{1}{4}$. |
Example #2
Determine the result of adding $7\frac{2}{5}$ and $6\frac{1}{3}$.
Solution
| Addition Process | Step-by-step Explanation |
| $7\frac{2}{5} + 6\frac{1}{3} =$ | Set up the addition process. |
| $7\frac{2}{5}$ ⟹ $\frac{37}{5}$ | Convert $7\frac{2}{5}$ to an improper fraction. |
| $6\frac{1}{3}$ ⟹ $\frac{19}{3}$ | Convert $6\frac{1}{3}$ to an improper fraction. |
| $\frac{37}{5} + \frac{19}{3} =$ | Set up the addition process. |
| $\frac{37}{5}$ ⟹ $\frac{111}{15}$ $\frac{19}{3}$ ⟹ $\frac{95}{15}$ | Find the least common denominator of 3 and 5. By prime factorization, the least common multiple of 3 and 5 is 15. Thus, we will divide the LCD to the original denominator and multiply the quotient to the numerator. |
| $\frac{111}{15}$ ⟹ $\frac{95}{15} =$ | Set up the addition process. |
| $\frac{111}{15}$ ⟹ $\frac{95}{15} =\frac{206}{}$ | Add the numerators. |
| $\frac{111}{15}$ ⟹ $\frac{95}{15} =\frac{206}{15}$ | Copy the denominator. |
| $\frac{206}{15}$ ⟹ $13\frac{11}{15}$ | Convert $\frac{206}{15}$ to a mixed number. |
| Therefore, the result of adding $7\frac{2}{5}$ and $6\frac{1}{3}$ is $13\frac{11}{15}$. |
How to subtract mixed numbers?
Subtraction is simply the opposite of addition. When subtracting mixed numbers, we always need to consider the minuends and the subtrahends and the denominators of the two.
Subtracting whole number and mixed number
We need to consider two cases if we are given a subtraction problem given a whole number and a mixed number.
Case #1: If the minuend is a mixed number
To subtract a whole number from a minuend, follow these steps:
- Subtract the given whole number from the whole number of the mixed number.
- Copy the fraction part of the mixed number on the final answer.
Example
What is the difference between $5\frac{6}{7}$ and 3?
Solution
| Subtraction Process | Step-by-step Explanation |
| $5\frac{6}{7} – 3 =$ | Set up the subtraction process. |
| $5\frac{6}{7} – 3 =2$ | Subtract the whole numbers. Hence, 5 – 3 = 2 |
| $5\frac{6}{7} – 3 = 2\frac{6}{7}$ | Copy the fraction part of the given in mixed numbers. |
| Therefore, the difference between $5\frac{6}{7}$ and 3 is $2\frac{6}{7}$. |
Case #2: If the subtrahend is a mixed number
To subtract a mixed number from a whole number, follow these steps.
- Convert the whole number to a fraction having the same denominator as the given mixed number.
- Convert the mixed number to an improper fraction.
- Subtract the numerators.
- Copy the denominator.
- Convert your final answer to a mixed number
Example
Find the result of subtracting $1\frac{1}{3}$ from 4.
Solution
| Subtraction Process | Step-by-step Explanation |
| $4 – 1\frac{1}{3} =$ | Set up the subtraction process. |
| 4 ⟹ $\frac{12}{3}$ | Convert 4 to an improper fraction. |
| $1\frac{1}{3}$ ⟹ $\frac{4}{3}$ | Convert $1\frac{1}{3}$ to an improper fraction. |
| $\frac{12}{3} – \frac{4}{3} = \frac{8}{}$ | Subtract the numerators. |
| $\frac{12}{3} – \frac{4}{3} = \frac{8}{3}$ | Copy the denominator. |
| $\frac{8}{3}$ ⟹ $2\frac{2}{3}$ | Convert $\frac{8}{3}$ to a mixed number. |
| Therefore, the result of subtracting $1\frac{1}{3}$ from 4 is $2\frac{2}{3}$. |
Subtracting mixed numbers with the same denominator
To subtract mixed numbers with the same denominators, follow these steps:
- Convert the mixed number to an improper fraction.
- Subtract the numerators.
- Copy the denominator.
- Convert the improper fraction to a mixed number.
Example #1
What is the difference between $3\frac{3}{8}$ and $\frac{5}{8}$?
Solution
| Subtraction Process | Step-by-step Explanation |
| $3\frac{3}{8} – \frac{5}{8} =$ | Set up the subtraction process. |
| $3\frac{3}{8}$ ⟹ $\frac{27}{8}$ | Convert $3\frac{3}{8}$ to an improper fraction. |
| $\frac{27}{8} – \frac{5}{8} = \frac{22}{}$ | Subtract the numerators. |
| $\frac{27}{8} – \frac{5}{8} = \frac{22}{8}$ | Copy the denominator. |
| $\frac{22}{8}$ ⟹ $2\frac{6}{8}$ | Convert $\frac{22}{8}$ to a mixed number. |
| $2\frac{6}{8}$ ⟹ $2\frac{3}{4}$ | Get the lowest term of $\frac{6}{8}$. |
| Therefore, the difference is $2\frac{3}{4}$. |
Example #2
Find the difference of subtracting $3\frac{2}{9}$ from $5\frac{4}{9}$.
Solution
| Subtraction Process | Step-by-step Explanation |
| $5\frac{4}{9} – 3\frac{2}{9} =$ | Set up the subtraction process. |
| $5\frac{4}{9}$ ⟹ $\frac{49}{9}$ | Convert $5\frac{4}{9}$ to an improper fraction. |
| $3\frac{2}{9}$ ⟹ $\frac{29}{9}$ | Convert $3\frac{2}{9}$ to an improper fraction. |
| $\frac{49}{9} – \frac{29}{9} = \frac{20}{}$ | Subtract the numerators. |
| $\frac{49}{9} – \frac{29}{9} = \frac{20}{9}$ | Copy the denominator. |
| $\frac{20}{9}⟹ 2\frac{2}{9}$ | Convert $\frac{20}{9}$ to a mixed number. |
| Therefore, the difference is $2\frac{2}{9}$. |
Example #3
What is the result of subtracting $\frac{23}{11}$ from $6\frac{2}{11}$?
Solution
| Subtraction Process | Step-by-step Explanation |
| $6\frac{2}{11} – \frac{23}{11} =$ | Set up the subtraction process. |
| $6\frac{2}{11}$ ⟹ $\frac{68}{11}$ | Convert $6\frac{2}{11}$ to an improper fraction. |
| $\frac{68}{11} – \frac{23}{11} = \frac{45}{}$ | Subtract the numerators. |
| $\frac{68}{11} – \frac{23}{11} = \frac{45}{11}$ | Copy the denominator. |
| $\frac{45}{11}$ ⟹ $4\frac{1}{11}$ | Convert $\frac{45}{11}$ to a mixed number. |
| Therefore, the result of subtracting $\frac{23}{11}$ from $6\frac{2}{11}$ is $4\frac{1}{11}$. |
Subtracting mixed numbers with different denominators
To subtract mixed numbers with different denominators, follow these steps:
- Convert the mixed number to an improper fraction.
- Find the least common denominator (LCD).
- Divide the LCD by the original denominators of the given mixed numbers.
- Multiply the quotient to the denominator of the improper fraction.
- Write the fractions based on the LCD.
- Subtract the numerators.
- Copy the LCD.
- Convert the improper fraction to a mixed number.
Example #1
What is the difference between $9\frac{3}{4}$ and $\frac{2}{3}$?
Solution
| Subtraction Process | Step-by-step Explanation |
| $9\frac{3}{4} – \frac{2}{3} =$ | Set up the subtraction process |
| $9\frac{3}{4}$ ⟹ $\frac{39}{4}$ | Convert $9\frac{3}{4}$ to an improper fraction. |
| $\frac{39}{4} – \frac{2}{3} =$ | Set up subtraction process. |
| $\frac{39}{4}$ ⟹ $\frac{117}{12}$ $\frac{2}{3}$ ⟹ $\frac{8}{12}$ | Find the least common denominator of 4 and 3. By prime factorization, the least common multiple of 4 and 3 is 12. Hence, we will divide the LCD to the original denominator and multiply the quotient to the numerator. |
| $\frac{117}{12} – \frac{8}{12} =$ | Set up the subtraction process. |
| $\frac{117}{12} – \frac{8}{12} = \frac{109}{}$ | Subtract the numerators. |
| $\frac{117}{12} – \frac{8}{12} = \frac{109}{12}$ | Copy the denominator. |
| $\frac{109}{12}$ ⟹ $9\frac{11}{2}$ | Convert $\frac{109}{12}$ to a mixed number. |
| Therefore, the difference is $9\frac{1}{12}$. |
Example #2
Determine the result of subtracting $3\frac{1}{10}$ from $8\frac{2}{5}$.
Solution
| Subtraction Process | Step-by-step Explanation |
| $8\frac{2}{5} – 3\frac{1}{10}$ | Set up the subtraction process |
| $8\frac{2}{5}$ ⟹ 425 | Convert $8\frac{2}{5}$ to an improper fraction. |
| $3\frac{1}{10}$ ⟹ $\frac{31}{10}$ | Convert $3\frac{1}{10}$ to an improper fraction. |
| $\frac{42}{5} – \frac{31}{10} =$ | Set up subtraction process. |
| $\frac{42}{5}$ ⟹ $\frac{84}{10}$ $\frac{31}{10}$ ⟹ $\frac{31}{10}$ | Find the least common denominator of 5 and 10. By prime factorization, the least common multiple of 5 and 10 is 10. Hence, we will divide the LCD to the original denominator and multiply the quotient to the numerator. |
| $\frac{84}{10} – \frac{31}{10} =$ | Set up the subtraction process. |
| $\frac{84}{10} – \frac{31}{10} =\frac{53}{}$ | Subtract the numerators. |
| $\frac{84}{10} – \frac{31}{10} =\frac{53}{10}$ | Copy the denominator. |
| $\frac{53}{10}$ ⟹ $5\frac{3}{10}$ | Convert $\frac{53}{10}$ to a mixed number. |
| Therefore, the difference is $5\frac{3}{10}$. |
Recommended Worksheets
Mixed Numbers (Mother’s Day Themed)
Subtraction of Mixed Numbers (Farm Themed) Worksheets
Addition of Mixed Numbers (Ages 10-11) Worksheets (Aviation themed)









